In this problem, you will demonstrate that the Gaussian PDF in Equation ( 9.64) is in fact the solution to the diffusion Equation ( 9.63). To do this, we will use frequency domain
methods. Define
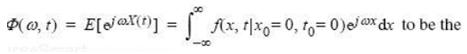time- varying characteristic function of the random process X (t).
(a) Starting from the diffusion Equation (9.63), show that the characteristic function must satisfy
Also, determine the appropriate initial condition for this differential equation. That is, find Φ (ω, t).
(b) Solve the first- order differential equation in part (a) and show that the characteristic function is of the form
(c) From the characteristic function, find the resulting PDF given by Equation (9.62).

D(0, t) = E[o@X()] Ax, txo=D0, to=0)e/ax dx to be the