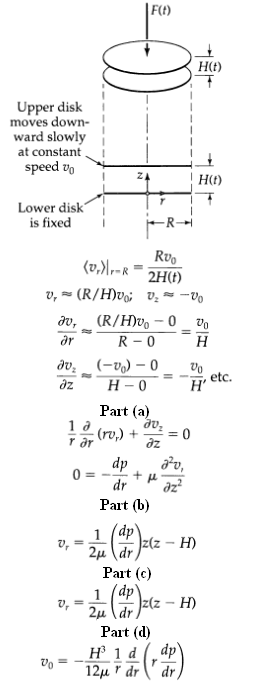
Parallel-disk compression viscometer 6 (Fig. 3C-1) A fluid fills completely the region between two circular disks of
Question:
Parallel-disk compression viscometer 6 (Fig. 3C-1) A fluid fills completely the region between two circular disks of radius R. The bottom disk is fixed, and the upper disk is made to approach the lower one very slowly with a constant speed v0, starting from a height H0 (and H0 < < R). The instantaneous height of the upper disk is H(t). It is desired to find the force needed to maintain the speed v0.
This problem is inherently a rather complicated unsteady-state flow problem. However, a useful approximate solution can be obtained by making two simplifications in the equations of change: (i) we assume that the speed v0 is so slow that all terms containing time derivatives can be omitted; this is the so-called "quasi-steady-state" assumption; (ii) we use the fact that H0 < < R to neglect quite a few terms in the equations of change by order-of-magnitude arguments. Note that the rate of decrease of the fluid volume between the disks is πR2v0 and that this must equal the rate of outflow from between the disks, which is 2πRH[r+R. Hence we now argue that vr(r, z) will be of the order of magnitude of r-R and that Vz(r, z) is of the order of magnitude of v0, so that and hence |vz| << vr.. We may now estimate the order of magnitude of various derivatives as follows: as r goes from 0 to R, the radial velocity v,. goes from zero to approximately (R/H)vo. By this kind of reasoning we get
(a) By the above-outlined order-of-magnitude analysis, show that the continuity equation and the r-component of the equation of motion become (with gz neglected) with the boundary conditions
B.C.1: at z = 0, vr = 0, vz = 0
B.C.2: at z = H(t), vr = 0, vz = – v0
B.C.3: at r = R, p = patm
(b) From Eqs. 3C.1-7 to 9 obtains.
(c) Integrate Eq. 3C.1-6 with respect to z and substitute the result from Eq. 3.C.1-11 to get
(d) Solve Eq. 3C.1-12 to get the pressure distribution
(e) Integrate [(p + τzz) - Patm] over the moving-disk surface to find the total force needed to maintain the disk motion: F(t) = 3πμv0R4/2[H(t)]3 this result can be used to obtain the viscosity from the force and velocity measurements.
(f) Repeat the analysis for a viscometer that is operated in such a way that a centered, circular glob of liquid never completely fills the space between the two plates. Let the volume of the sample be V and obtain F(t) = 3μv0V2/2π[H(t)]5
(g) Repeat the analysis for a viscometer that is operated with constant applied force, F0. The viscosity is then to be determined by measuring H as a function of time, and the upper-plate velocity is not a constant. Show that 1/[H(t)]2 = 1/H20 + 4F0t/3πμR4
Step by Step Answer:
Paralleldisk compression viscometer a The equation of continuity of Eq B42 for incompressible fluids ...View the full answer
