The part of the decomposition of G in Theorem 11.12 corresponding to the subgroups of prime-power order can also be written in the form Z_m1 x Zm₂ x · · · x Z_mr where m_i divides m_i+1 for i = 1, 2, · · ·, r - 1. The numbers m_i can be shown to be unique, and are the torsion coefficients of G. 

a. Find the torsion coefficients of Z₄ x Z₉ . 

b. Find the torsion coefficients of Z₆ x Z₁₂ x Z₂₀ . 

c. Describe an algorithm to find the torsion coefficients of a direct product of cyclic groups.

Data from Theorem 11.12

(Fundamental Theorem of Finitely Generated Abelian Groups) Every finitely generated abelian group G is isomorphic to a direct product of cyclic groups in the for

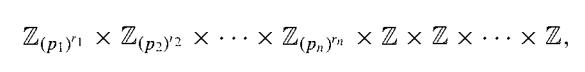
where the p_i are primes, not necessarily distinct, and the r_i are positive integers. The direct product is unique except for possible rearrangement of the factors; that is, the number (Betti number of G) of factors Z is unique and the prime powers (p_i )^ri are unique.

Transcribed Image Text:

Z(p₁)'¹ X Z(p2)'² X X Z(p) x Zxzx. x Z,