In this exercise, we use the notation of the proof of Theorem 1 and prove
\[
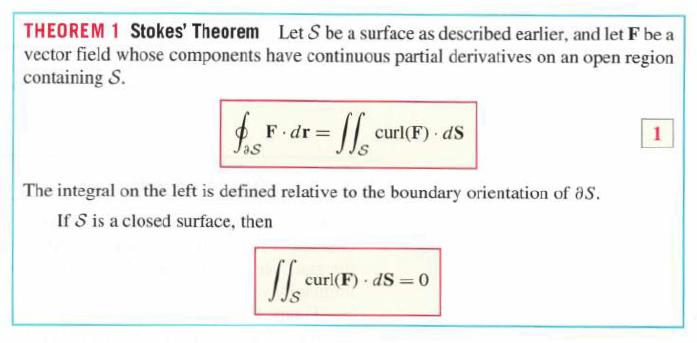\oint_{C} F_{3}(x, y, z) \mathbf{k} \cdot d \mathbf{r}=\iint_{\mathcal{S}} \operatorname{curl}\left(F_{3}(x, y, z) \mathbf{k}ight) \cdot d \mathbf{S}
\]
In particular, \(\mathcal{S}\) is the graph of \(z=f(x, y)\) over a domain \(\mathcal{D}\), and \(\mathcal{C}\) is the boundary of \(\mathcal{S}\) with parametrization \((x(t), y(t), f(x(t), y(t)))\).
(a) Use the Chain Rule to show that
\[
F_{3}(x, y, z) \mathbf{k} \cdot d \mathbf{r}=F_{3}\left(x(t), y(t), f(x(t), y(t))\left(f_{x}(x(t), y(t)) x^{\prime}(t)+f_{y}(x(t), y(t)) y^{\prime}(t)ight) d tight.
\]
and verify that
\[
\oint_{C} F_{3}(x, y, z) \mathbf{k} \cdot d \mathbf{r}=\oint_{C_{0}}\left\langle F_{3}(x, y, z) f_{x}(x, y), F_{3}(x, y, z) f_{y}(x, y)ightangle \cdot d \mathbf{r}
\]
where \(C_{0}\) has parametrization \((x(t), y(t))\).
(b) Apply Green's Theorem to the line integral over \(C_{0}\) and show that the result is equal to the right-hand side of Eq. (10).

\[
\oint_{C} F_{3}(x, y, z) \mathbf{k} \cdot d \mathbf{r}=\iint_{\mathcal{S}} \operatorname{curl}\left(F_{3}(x, y, z) \mathbf{k}ight) \cdot d \mathbf{S}
\]

THEOREM 1 Stokes' Theorem Let S be a surface as described earlier, and let F be a vector field whose components have continuous partial derivatives on an open region containing S. & F. dr = = curl(F). dS The integral on the left is defined relative to the boundary orientation of S. If S is a closed surface, then Js curl(F). ds = 0 1