Suppose that the curve \(C\) in Figure 7 has the polar equation \(r=f(\theta)\).
(a) Show that \(\mathbf{r}(\theta)=\langle f(\theta) \cos \theta, f(\theta) \sin \thetaangle\) is a counterclockwise parametrization of \(C\).
(b) In Section 11.4, we showed that the area of the region \(\mathcal{R}\) is given by the formula
\[
\text { area of } \mathcal{R}=\frac{1}{2} \int_{\alpha}^{\beta} f(\theta)^{2} d \theta
\]
Use the result of Exercise 37 to give a new proof of this formula. Evaluate the line integral in Eq. (1) using \(\mathbf{r}(\theta)\).

Data From Exercise 37

In Section 17.1, we showed that if \(C\) is a simple closed curve, oriented counterclockwise, then the area enclosed by \(C\) is given by
\[
\text { area enclosed by } C=\frac{1}{2} \oint_{C} x d y-y d x
\]
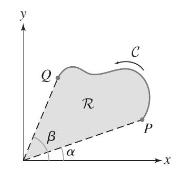
Suppose that \(C\) is a path from \(P\) to \(Q\) that is not closed but has the property that every line through the origin intersects \(C\) in at most one point, as in Figure 7. Let \(\mathcal{R}\) be the region enclosed by \(C\) and the two radial segments joining \(P\) and \(Q\) to the origin. Show that the line integral in Eq. (1) is equal to the area of \(\mathcal{R}\). Show that the line integral of \(\mathbf{F}=\langle-y, xangle\) along the two radial segments is zero and apply Green's Theorem.

Transcribed Image Text:

THEOREM 1 Green's Theorem Let D be a domain whose boundary D is a simple closed curve, oriented counterclockwise. If F and F have continuous partial deriva- tives in an open region containing D, then F2 OF - F2dy = f ( 312 F) d A x dy $ Fidx + aD 2