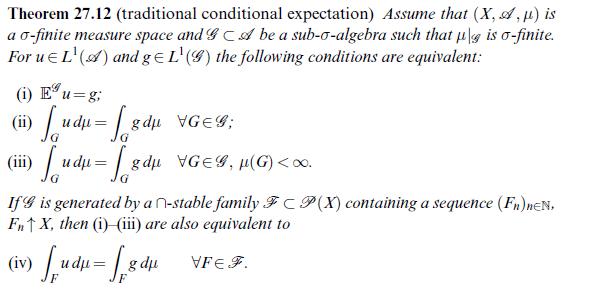
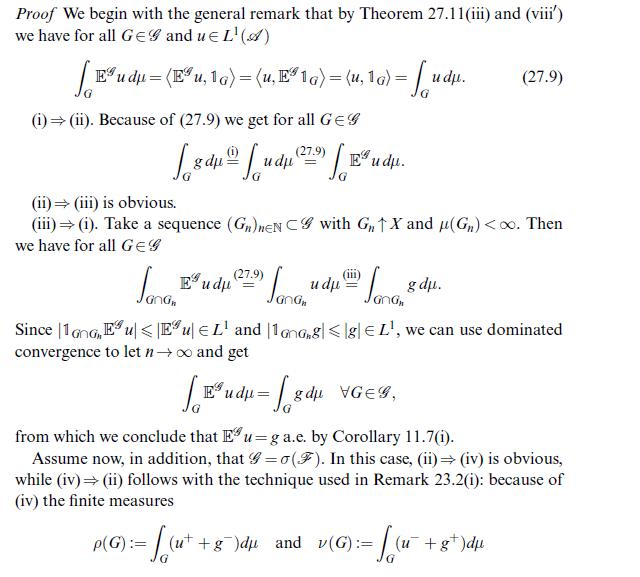
Conditional expectations. Let \((X, \mathscr{A}, \mu)\) be a \(\sigma\)-finite measure space and \(\mathscr{F} \subset \mathscr{A}\) be a sub\(\sigma\)-algebra. Then use the Radon-Nikodým theorem to show that for every \(u \in \mathcal{L}^{1}(\mathscr{A})\) there exists a - unique up to null sets - \(\mathscr{F}\)-measurable function \(u^{\mathscr{F}} \in \mathcal{L}^{1}(\mathscr{F})\) such that

\[\int_{F} u^{\mathscr{F}} d \mu=\int_{F} u d \mu \quad \forall F \in \mathscr{F} .\]

Use this result to rephrase the (sub, super)martingale property 23.1 .

Remark. Since \(u^{\mathscr{F}}\) is unique (modulo null sets) one often writes \(u^{\mathscr{F}}=E^{\mathscr{F}} u\), where \(E^{\mathscr{F}}\) is an operator which is called conditional expectation. We will introduce this operator in a different way in Chapter 27 and show in Theorem 27.12 that we could have defined \(E^{\mathscr{F}}\) by (25.21). 

Data from theorem 27.12

Transcribed Image Text:

Theorem 27.12 (traditional conditional expectation) Assume that (X, A,p) is a o-finite measure space and GCA be a sub-o-algebra such that plg is o-finite. For u E L (A) and gEL' (9) the following conditions are equivalent: (i) Eu=g; (ii) [ud = [gd du VGE9; (iii) [ = 8 +9 (G) < . u g du If is generated by an-stable family FP(X) containing a sequence (Fn)neN, FnX, then (1)-(iii) are also equivalent to VFEF. (iv) Ludu= [8 d g