Stieltjes measure (2). Stieltjes integrals. This continues Problem 6.1. Let (mu) and (u) be two measures on
Question:
Stieltjes measure (2). Stieltjes integrals. This continues Problem 6.1. Let \(\mu\) and \(u\) be two measures on \((\mathbb{R}, \mathscr{B}(\mathbb{R}))\) such that \(\mu((-n, n]), u((-n, n])
![if x > 0, if x = 0, -(x, 0], if x <0, (0, x], F(x):= 0, and G(x):= if x > 0, if x=0, -v(x, 0], if x <0, v(0,](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1705/7/3/9/38265ab847695cab1705739381868.jpg)
the associated right-continuous distribution functions (in Problem 6.1 we considered leftcontinuous distribution functions). Moreover, set \(\Delta F(x)=F(x)-F(x-)\) and \(\Delta G(x)=\) \(G(x)-G(x-)\).
(i) Show that \(F, G\) are increasing and right-continuous, and that \(\Delta F(x)=0\) if, and only if, \(\mu\{x\}=0\). Moreover, \(F\) and \(\mu\) are in one-to-one correspondence.
(ii) Since measures and distribution functions are in one-to-one correspondence, it is customary to write \(\int u d \mu=\int u d F\), etc.
If \(a
\[\mu \times u(B)=\int_{(a, b]} F(s) d G(s)-F(a)(G(b)-G(a)) .\]
(iii) Integration by parts. Show that
\[
\begin{aligned}
& F(b) G(b)-F(a) G(a) \\
& \quad=\int_{(a, b]} F(s) d G(s)+\int_{(a, b]} G(s-) d F(s) \\
& \quad=\int_{(a, b]} F(s-) d G(s)+\int_{(a, b]} G(s-) d F(s)+\sum_{a\end{aligned}
\]
[expand \(\mu \times u\left((a, b]^{2}ight)\) in two different ways, using (ii). Note that the series appearing in the formula is at most countable because of Lemma 14.14 .]
(iv) Change of variable formula. Let \(\phi\) be a \(C^{1}\)-function. Then
![o(F(b)) (F(a)) = ['(F(s-))dF(s) + [(F(s)) (F(s-)) 6'(F(s))AF(s)]. - a](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1705/7/3/9/47765ab84d5a1e491705739476905.jpg)
[ use (iii) to show the change-of-variable formula for polynomials and then use the fact that continuous functions can be uniformly approximated by a sequence of polynomials - see Weierstraß' approximation theorem, Theorem 28.6 .]
Data from problem 6.1
Stieltjes measure (1).
(i) Let \(\mu\) be a measure on \((\mathbb{R}, \mathscr{B}(\mathbb{R}))\) such that \(\mu[-n, n)
\[
F_{\mu}(x):= \begin{cases}\mu[0, x) & \text { if } x>0 \\ 0 & \text { if } x=0 \\ -\mu[x, 0) & \text { if } x\]
is a monotonically increasing and left-continuous function \(F_{\mu}: \mathbb{R} ightarrow \mathbb{R}\).
Remark. Increasing and left-continuous functions are called Stieltjes functions.
(ii) Let \(F: \mathbb{R} ightarrow \mathbb{R}\) be a Stieltjes function (see part (i)). Show that
\[
u_{F}([a, b)):=F(b)-F(a), \quad \forall a, b \in \mathbb{R}, a\]
has a unique extension to a measure on \(\mathscr{B}(\mathbb{R})\).
[ check the assumptions of Theorem 6.1 with \(\mathcal{S}=\{[a, b): a \leqslant b\}\).]
(iii) Conclude that for every measure \(\mu\) on \((\mathbb{R}, \mathscr{B}(\mathbb{R})\) ) satisfying \(\mu[-r, r)0\), there is some Stieltjes function \(F=F_{\mu}\) such that \(\mu=u_{F}\) (as in part (ii)).
(iv) Which Stieltjes function \(F\) corresponds to \(\lambda\) (one-dimensional Lebesgue measure)?
(v) Which Stieltjes function \(F\) corresponds to \(\delta_{0}\) (Dirac measure on \(\mathbb{R}\) )?
(vi) Show that \(F_{\mu}\) as in part (i) is continuous at \(x \in \mathbb{R}\) if, and only if, \(\mu\{x\}=0\).
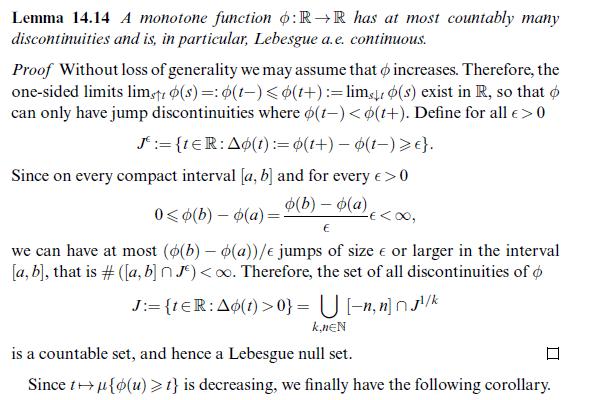
Data from lemma 14.14
Data from theorem 28.6
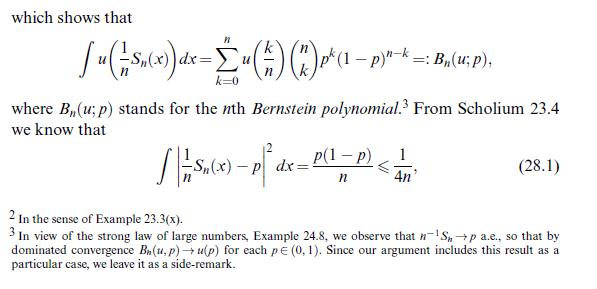
![Theorem 28.6 (Weierstra) Polynomials are dense in C[0, 1] w.rt. uniform convergence. Proof (S. N. Bernstein)](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1705/7/4/0/96565ab8aa5846b31705740964695.jpg)
![since the function p-p(1-p) attains its maximum at p= 1/2. As ue C[0, 1] is uniformly continuous, u(x)-u(y) |](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1705/7/4/0/99265ab8ac048d891705740990096.jpg)
Step by Step Answer:






