Let (left(B_{t} ight)_{t geqslant 0}) be a (mathrm{BM}^{1}) and (tau) some a.s. finite stopping time such that
Question:
Let \(\left(B_{t}\right)_{t \geqslant 0}\) be a \(\mathrm{BM}^{1}\) and \(\tau\) some a.s. finite stopping time such that \(\left|B_{t \wedge \tau}\right|, t \geqslant 0\), is bounded. Show that \(\mathbb{E}\left(\tau^{2}\right) \leqslant 4 \mathbb{E}\left(B_{\tau}^{4}\right)\). Conclude from this that we have in Theorem 14.2, and Corollary 14.3, "for free" the bounds \(\mathbb{E}\left(\tau^{2}\right) \leqslant 4 \mathbb{E}\left(X^{4}\right)\), and \(\mathbb{E}\left(\left(\tau_{n}-\tau_{n-1}\right)^{2}\right) \leqslant 4 \mathbb{E}\left(X_{1}^{4}\right)\), respectively. (These latter bounds are understood in \([0, \infty]\), though).
a) Use a suitable Brownian martingale involving \(B_{t}^{4}\).
b) Look at the last part of the proof of Theorem 14.2.
Data From 14.2 Theorem
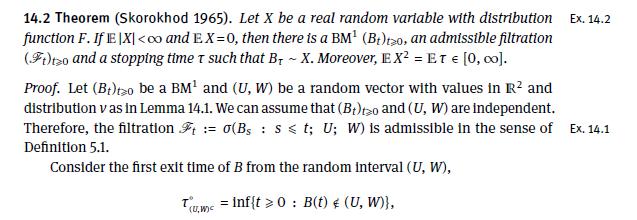
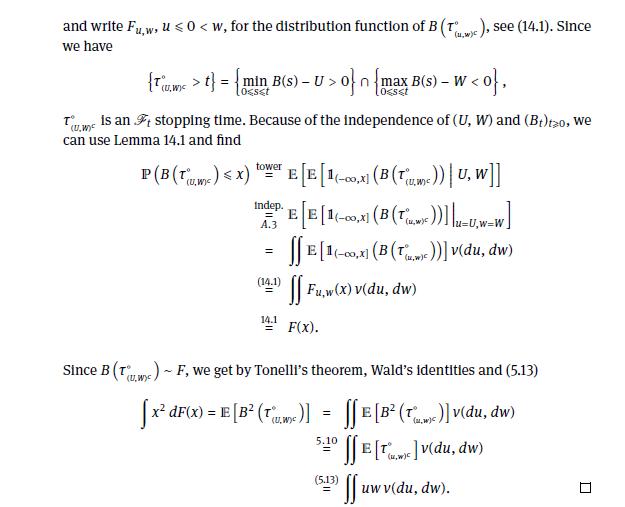
Data From14.3 Corollary
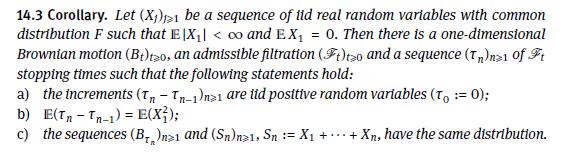
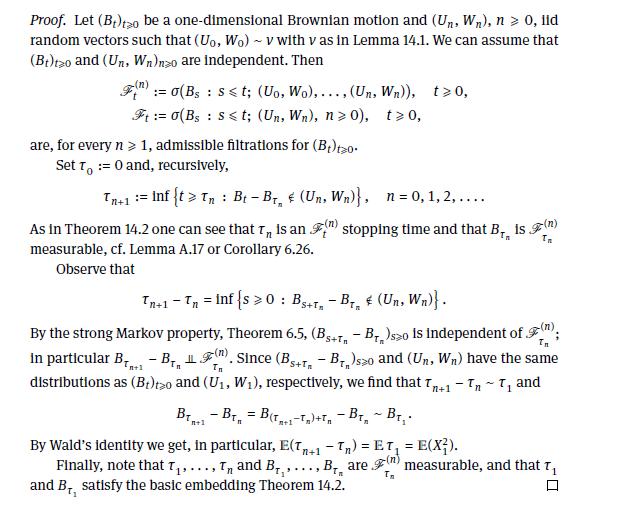
Step by Step Answer:

Brownian Motion A Guide To Random Processes And Stochastic Calculus De Gruyter Textbook
ISBN: 9783110741254
3rd Edition
Authors: René L. Schilling, Björn Böttcher





