Let (left(B_{t} ight)_{t geqslant 0}) be a (mathrm{BM}^{d}) and set (u(t, x):=P_{t} u(x)). Adapt the proof of
Question:
Let \(\left(B_{t}\right)_{t \geqslant 0}\) be a \(\mathrm{BM}^{d}\) and set \(u(t, x):=P_{t} u(x)\). Adapt the proof of Proposition 7.3.g) and show that for \(u \in \mathcal{B}_{b}\left(\mathbb{R}^{d}\right)\) the function \(u(t, \cdot) \in \mathcal{C}^{\infty}\) and \(u \in \mathcal{C}^{1,2}\) (i.e. once continuously differentiable in \(t\), twice continuously differentiable in \(x\) ).
Data From Proposition 7.3
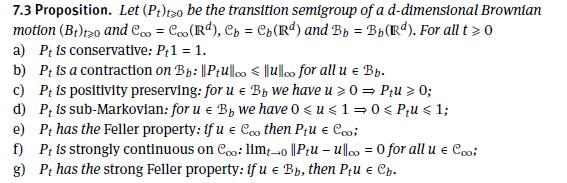
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Brownian Motion A Guide To Random Processes And Stochastic Calculus De Gruyter Textbook
ISBN: 9783110741254
3rd Edition
Authors: René L. Schilling, Björn Böttcher
Question Posted:





