Answered step by step
Verified Expert Solution
Question
1 Approved Answer
3. Finding a hidden slope. Let p be a prime number. Suppose you are given a black-box function f: {0, 1,. ,p 1} x
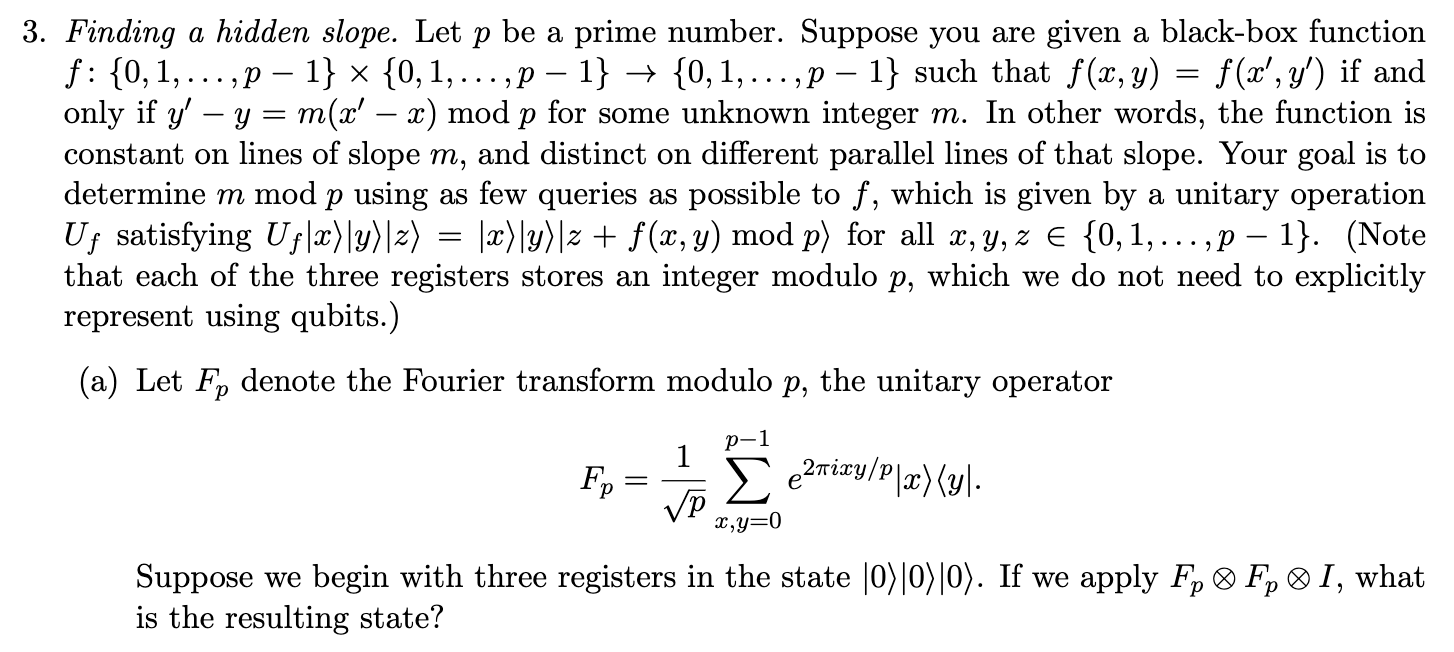
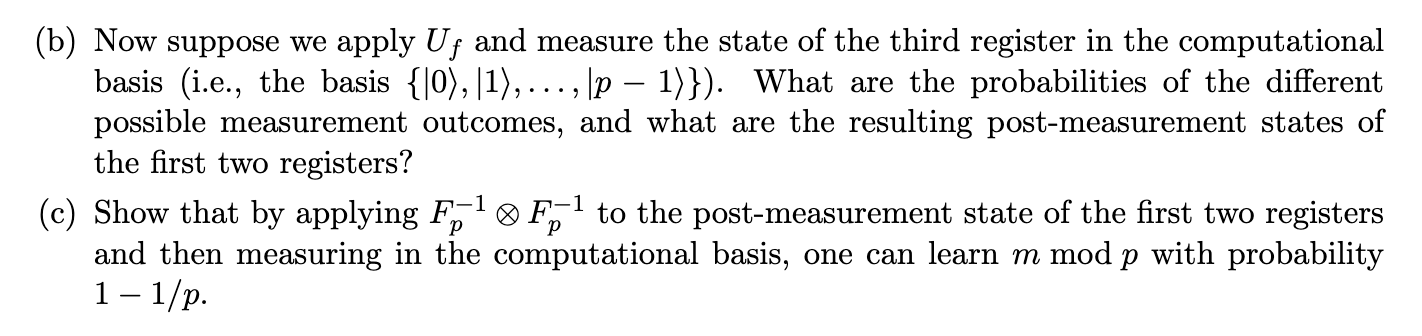
3. Finding a hidden slope. Let p be a prime number. Suppose you are given a black-box function f: {0, 1,. ,p 1} x {0, 1, .. ..p 1} {0, 1,...,p - 1} such that f(x,y) f(x', y') if and only if y' y = m(x x) mod p for some unknown integer m. In other words, the function is constant on lines of slope m, and distinct on different parallel lines of that slope. Your goal is to determine m mod p using as few queries as possible to f, which is given by a unitary operation Uf satisfying Uf|x)|y)|z) = |x)|y)|z + f(x, y) mod p) for all x, y, z {0, 1,...,p 1}. (Note that each of the three registers stores an integer modulo p, which we do not need to explicitly represent using qubits.) - (a) Let Fp denote the Fourier transform modulo p, the unitary operator p-1 2nixy/P\x) (y\. Fp = P = x,y=0 Suppose we begin with three registers in the state |0)|0)|0). If we apply Fp Fp I, what is the resulting state? (b) Now suppose we apply U and measure the state of the third register in the computational basis (i.e., the basis {|0), 1),..., p - 1)}). What are the probabilities of the different possible measurement outcomes, and what are the resulting post-measurement states of the first two registers? 1 (c) Show that by applying F F to the post-measurement state of the first two registers and then measuring in the computational basis, one can learn m mod p with probability 1-1/p. 3. Finding a hidden slope. Let p be a prime number. Suppose you are given a black-box function ..p 1} {0, 1,. .. ,p - 1} such that f(x, y) f(x', y') if and f: {0, 1,. ,p - 1} x {0, 1, .. only if y' y = m(x' x) mod p for some unknown integer m. In other words, the function is constant on lines of slope m, and distinct on different parallel lines of that slope. Your goal is to determine m mod p using as few queries as possible to f, which is given by a unitary operation Uf satisfying Uf|x)|y)|z) = |x)|y)]z + f(x, y) mod p) for all x, y, z {0, 1, ..., p 1}. (Note that each of the three registers stores an integer modulo p, which we do not need to explicitly represent using qubits.) - (a) Let Fp denote the Fourier transform modulo p, the unitary operator p-1 2nixy/P\x) (y\. Fp = P = x,y=0 Suppose we begin with three registers in the state |0)|0)|0). If we apply Fp Fp I, what is the resulting state? (b) Now suppose we apply U and measure the state of the third register in the computational basis (i.e., the basis {|0), 1),..., p - 1)}). What are the probabilities of the different possible measurement outcomes, and what are the resulting post-measurement states of the first two registers? 1 (c) Show that by applying F0 F to the post-measurement state of the first two registers and then measuring in the computational basis, one can learn m mod p with probability 1-1/p.
Step by Step Solution
★★★★★
3.34 Rating (151 Votes )
There are 3 Steps involved in it
Step: 1
The problem presented is a quantum computing problem that utilizes the concept of the Quantum Fourier Transform QFT and is related to the Hidden Subgroup Problem a general framework for several quantu...
Get Instant Access to Expert-Tailored Solutions
See step-by-step solutions with expert insights and AI powered tools for academic success
Step: 2

Step: 3

Ace Your Homework with AI
Get the answers you need in no time with our AI-driven, step-by-step assistance
Get Started


