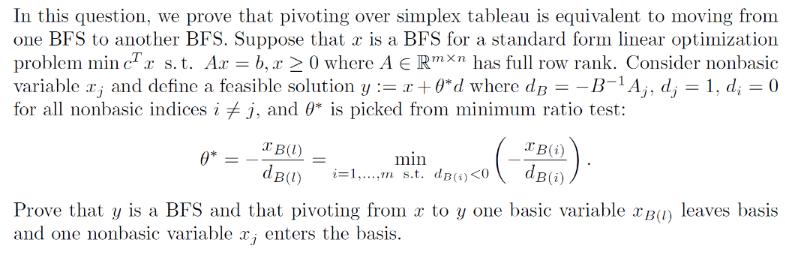
In this question, we prove that pivoting over simplex tableau is equivalent to moving from one BFS to another BFS. Suppose that a is a BFS for a standard form linear optimization problem min er s. t. Ar=b, r20 where A Rmxn has full row rank. Consider nonbasic variable , and define a feasible solution y := x+0*d where dB = -B-Aj, dj = 1, di = 0 for all nonbasic indices i #j, and 0* is picked from minimum ratio test: XB(1) dB(1) min i=1.....m s.t. dB(0) 50- JB(i) dB(), Prove that y is a BFS and that pivoting from a to y one basic variable B(1) leaves basis and one nonbasic variable x, enters the basis.