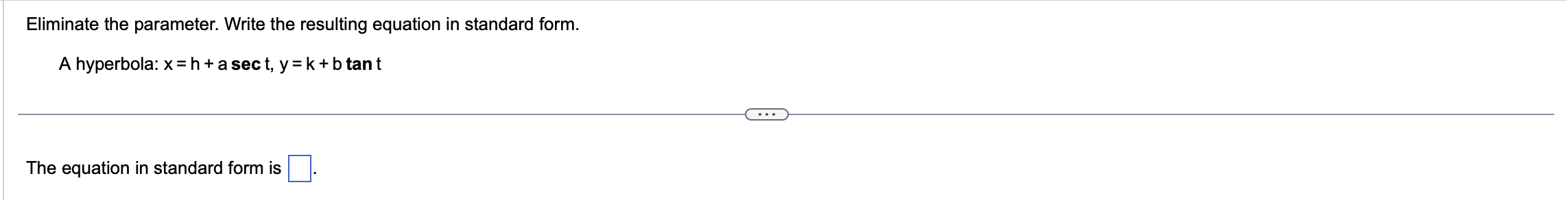
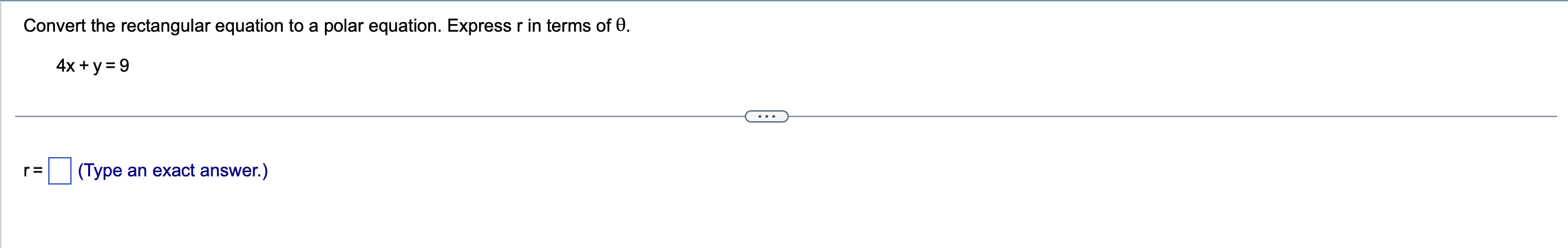
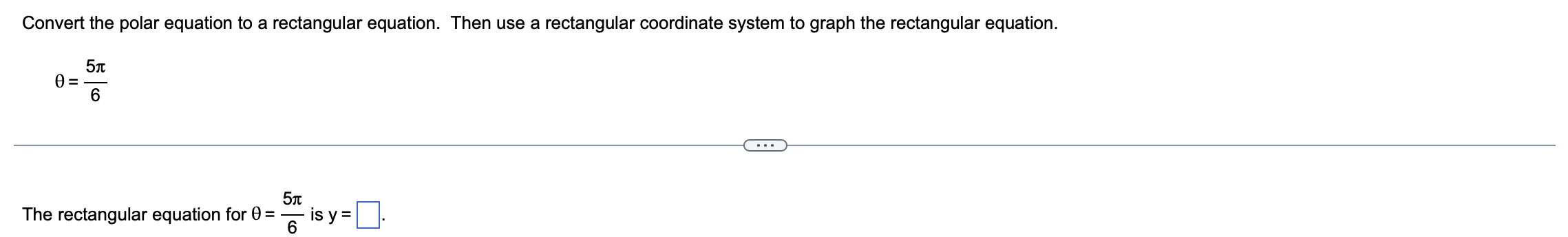
Eliminate the parameter. Write the resulting equation in standard form. A hyperbola: x = h + a sect, y=k+b tant The equation in standard form is The parametric equations of an ellipse are x = h + a cost and y=k+ b sint, where (h,k) is the center, a is the distance to the major axis points left and right of the center, and b is the distance to the minor axis points above and below the center. Find a set of parametric equations for an ellipse centered at (1,7) with vertices 5 units to the left and right of the center and endpoints of the minor axis 3 units above and below the center. The parametric equations for an ellipse are x = and y= The polar coordinates of a point are given. Find the rectangular coordinates of this point. 3 What are the rectangular coordinates of this point? (Type an ordered pair. Simplify your answer, including any radicals. Rationalize all denominators. Use integers or fractions for any numbers in the expression.) Convert the rectangular equation to a polar equation. Express r in terms of 0. 4x + y = 9 r= (Type an exact answer.) Convert the polar equation to a rectangular equation. Then use a rectangular coordinate system to graph the rectangular equation. 5x 0= 6 The rectangular equation for 0 = 5 6 is y = Complete the sentence below. Rose curves are characterized by equations of the form r = a cos (n) or r=a sin (n), a 0. If n0 is even, the rose has Rose curves are characterized by equations of the form r = a cos (n) or r = a sin (n), a 0. If n #0 is even, the rose has petals; if n 1 is odd, the rose has petals; if n 1 is odd, the rose has petals. petals. Convert the polar equation to a rectangular equation. Then use a rectangular coordinate system to graph the rectangular equation. r sin 20 = 8 The rectangular equation is y= 1 Write a polar equation for an ellipse which a has a focus at the pole, vertex at (12, 0) and e= . 6 Write a polar equation using the standard form r = 7 (Simplify your answer.) r= ep 1 - e cos 0