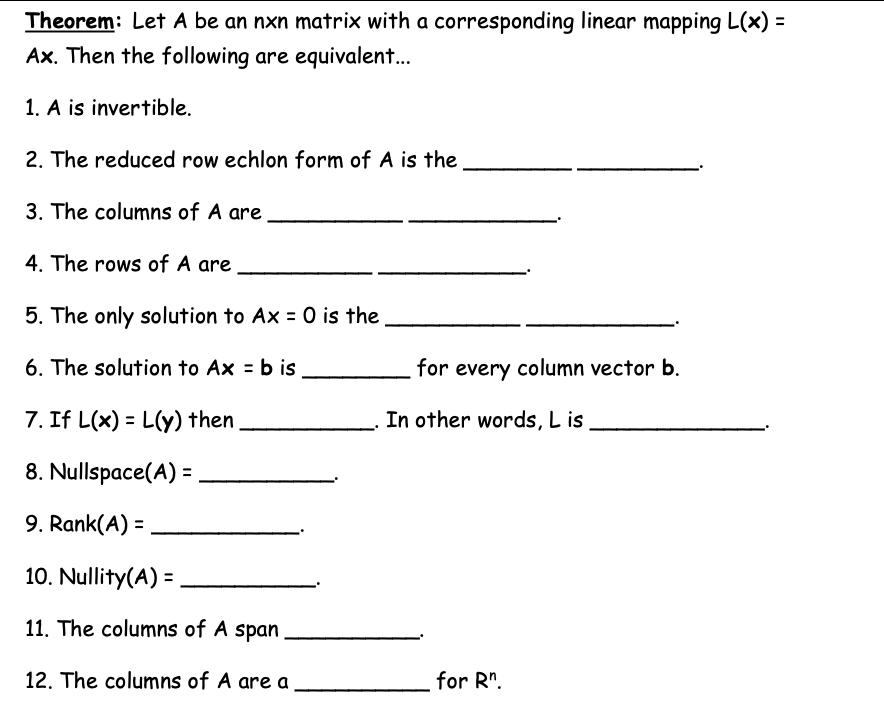
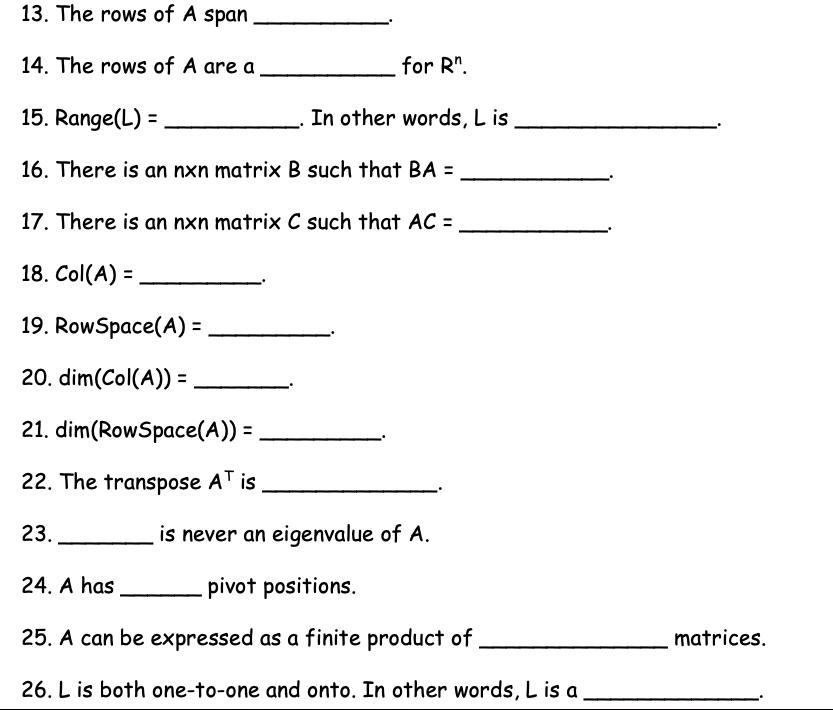
Theorem: Let A be an nxn matrix with a corresponding linear mapping L(x) = Ax. Then the following are equivalent... 1. A is invertible. 2. The reduced row echlon form of A is the 3. The columns of A are. 4. The rows of A are 5. The only solution to Ax = 0 is the. 6. The solution to Ax = b is 7. If L(x)=L(y) then 8. Nullspace(A) = 9. Rank(A) = 10. Nullity(A) = 11. The columns of A span 12. The columns of A are a for every column vector b. . In other words, L is for Rn. 13. The rows of A span 14. The rows of A are a 15. Range(L) = 16. There is an nxn matrix B such that BA = 18. Col(A) = 19. RowSpace(A) = 20. dim(Col(A)) = 21. dim(RowSpace(A)) = 22. The transpose AT is 17. There is an nxn matrix C such that AC = 23. for Rn. In other words, L is is never an eigenvalue of A. pivot positions. 25. A can be expressed as a finite product of 26. L is both one-to-one and onto. In other words, L is a 24. A has matrices. 27. For all matrices B and C, if AB = AC then. 28. For all matrices B and C, if BA= CA then 29. The determinant of A is 30. Can you come up with a 30th equivalent expression? Write yours below!