A liquid-phase chemical reaction A ? B takes place in a well-stirred tank. The concentration of A in the feed is C_A0 (mol/m³), and that in the tank and outlet stream is C_A (mol/m³). Neither concentration varies with time. The volume of the tank contents is V(m³) and the volumetric flow rate of the inlet and outlet streams is v (m³/s). The reaction rate (the rate at which A is consumed by reaction in the tank) is given by the expression r (mol A consumed/s) = kVC_A where k is a constant.

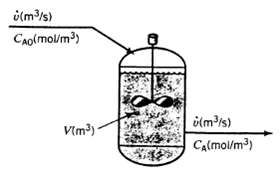
(a) Is this process continuous, batch, or semi batch? Is it transient or steady-state?

(b) What would you expect the reactant concentration C_A to equal if k = 0 (no reaction)? What should it approach if k ? ? (infinitely rapid reaction)?

(c) Write a differential balance on A, stating which terms in the general balance equation (accumulation = input + generation ? output ? consumption) you discarded and why you discarded them. Use the balance to derive the following relation between the inlet and outlet reactant concentrations: C_A = C_A0/1 + kV/v Verity that this relation predicts the results in part (b).

(m/s) Cao(mol/m3) V(m) (m/s) CA(mol/m)