Theorem 48.3 described conjugation isomorphisms for the case where a and were conjugate algebraic elements over
Question:
Theorem 48.3 described conjugation isomorphisms for the case where a and β were conjugate algebraic elements over F. Is there a similar isomorphism of F(α) with F(β) in the case that a and β are both transcendental over F?
Data from Theorem 48.3
Let F be a field, and let α and β be algebraic over F with deg(α, F) = n. The map ψα.β :F(α) → F(β) defined by ψα.β(c0 + ciα +· · ·+ Cn-1αn-¹) = c0 + c1β + · · ·+ Cn-1βn-1 for ci ∈ F is an isomorphism of F(α) onto F(β) if and only if a and are conjugate over F.
Proof Suppose that ψα.β : F(α) → F(β) as defined in the statement of the theorem is an isomorphism. Let irr(σ, F) = a0 + a1x + · · · + anxn. Then a0 + a1α + · · · + anαn = 0, so ψα.β(a0 + a1α + ... + anαn) = a0 + a1β + ... + anβn = 0.
By the last assertion in the statement of Theorem 29.13 this implies that irr(β, F) divides irr(α, F). A similar argument using the isomorphism (ψα.β)-1 = ψβ,α shows that irr(α, F) divides irr(β, F). Therefore, since both polynomials are monic, irr(α, F) = irr(β, F), so α and β are conjugate over F.
Conversely, suppose irr(α, F) = irr(β, F) = p(x). Then the evaluation homomorphisms ∅α: F[x] → F(α) and ∅β : F[x] → F(β) both have the same kernel (p(x)). By Theorem 26.17, corresponding to ∅α: F[x] → F(α), there is a natural isomorphism ψα mapping F[x]/(p(x)) onto ∅α:[F[x]] = F(α). Similarly, ∅β gives rise to an isomorphism ψβ mapping F[x]/(p(x)) onto F(β). Let ψα.β = ψβ(ψα)-1. These mappings are diagrammed in Fig. 48.4 where the dashed lines indicate corresponding elements under the mappings. As the composition of two isomorphisms,ψα.β is again an isomorphism and maps F(α) onto F(β). For (c0 + c1α + · · + cn-1an-1) ∈ F(α), we have
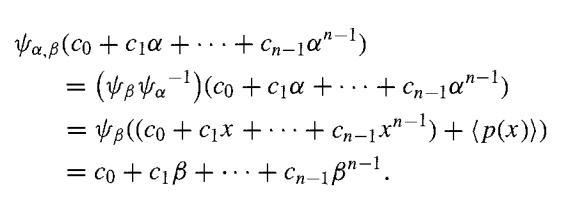
Thus ψα,β is the map defined in the statement of the theorem.
Step by Step Answer:
Yes if and are transcendentals over F then F F w...View the full answer
