FREE Trial

A can in the shape of a right circular cylinder is required to have a volume of 500 cubic centimeters.

Question:

A can in the shape of a right circular cylinder is required to have a volume of 500 cubic centimeters. The top and bottom are made of material that costs 60 per square centimeter, while the sides are made of material that costs 4¢ per square centimeter.

(a) Express the total cost C of the material as a function of the radius r of the cylinder. (Refer to Figure 43.)

Figure 43

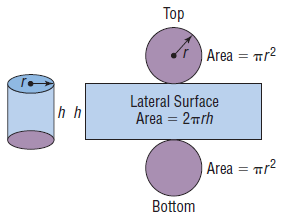
Top Area = mr2 Lateral Surface Area = 2mrh Area = r2 Bottom


(b) Graph C = C(r). For what value of r is the cost C a minimum?

Transcribed Image Text:

Top Area = mr2 Lateral Surface Area = 2mrh Area = r2 Bottom

This problem has been solved!


Do you need an answer to a question different from the above? Ask your question!

Step by Step Answer:

Answer rating: 66% (3 reviews)

a b Graphing Using MIN…View the full answer


answer-question
Answered By
tutor-profile-image
Mahesh G
I have more than 7 years of experience in teaching physics, mathematics and python programming to more than 600 students including both online and offline tutoring. I follow the following 7 step fundamental approach towards tutoring. 1. Curiosity, scope, enlightenment of the topic in hand. 2. Problem Definitions and elaboration. 3. Requisite mathematics, analytical abilities and quantitative aptitude. 4. Preparing Algorithms for problem statement. 5. Concepts with analogies and building algorithm. 6. Introspection and improvising. 7. Daily class wise Cheat sheets(its not cheating) for consolidation.
5.00+ 1+ Reviews 10+ Question Solved
Related Book For  answer-question
Precalculus

Precalculus

ISBN: 978-0321716835

9th edition

Authors: Michael Sullivan

See More Books
Question Details
Chapter # 4
Section: Section 4.3
Problem: 55
View Solution
Create a free account to access the answer
Cannot find your solution?
Post a FREE question now and get an answer within minutes. * Average response time.
Question Posted: January 10, 2019 06:25:45
See More Questions

Related Video

The area of a circle is given by the formula: A = ?r^2 Where A is the area, ? is a mathematical constant (approximately 3.14), and r is the radius of the circle. The radius is the distance from the center of the circle to the edge. So for example, if the radius of a circle is 5 units, the area would be: A = ?(5^2) = ?(25) = 78.5 square units Here three friends went out to a restaurant and decided to have pizza for lunch. While they were waiting for their order, one of them stepped out to take a call but promised to return in two minutes. The waiter came and presented them with the menu, and they decided to order two 10-inch pepperoni pizzas. The waiter took their order and left. Just then, the third friend returned and asked what they had ordered. They explained that they had ordered two small pepperoni pizzas as they were only three of them and it would be enough for them. However, the third friend suggested that for the same price, they could get a large pizza that would be much bigger than the two small ones. He asked the waiter for a pen and paper and proceeded to calculate the area of the two small pizzas and compared it to the area of one large pizza. The friends were amazed at how the circle measurements worked and decided to change their order from two small pizzas to one large pizza.

Students also viewed these Mathematics questions

Q:
A metal storage tank with volume V is to be constructed in the shape of a right circular cylinder surmounted by a hemisphere. What dimensions will require the least amount of metal?
A:
h 3V 5T S r 0 for r T 3V We minimize the surface area S r 2r......
Q:
An 18-oz jar of peanut butter in the shape of a right circular cylinder is 5 in. high and 3 in. in diameter and sells for $2.89. In the same store, a 28-oz jar of the same brand is 5 1/2 in. high and...
A:
Let V represent the volume and p represent the price of a......
Q:
A storage can is to be made in the shape of a right circular cylinder of height h and radius r. Find the surface area of the container (including the circular top and bottom) as a function of r only,...
A:
S 2r 2 2rh Since the volume is to be 8......
Q:
Conduct online research for federal income tax brackets for the current year. Which tax bracket do you fit into for your gross household income? How close is your gross household income to the next...
A:
Taxable income The United States federal income tax has seven significant tax brackets that include 10 15 25 28 33 35 and 39 6 for instance the amount of tax you pay depends on individuals filing stat......
Q:
Net Play Company uses a job order cost system in each of its three manufacturing departments. Manufacturing overhead is applied to jobs on the basis of direct labour cost in Department A, direct...
A:
a Department A 720 000 600 000 120 of direct labour......
Q:
Uncle Harry and Aunt Minnie will both be attending your next family reunion. Unfortunately, they hate each other. Unless they are seated with at least two people between them, they are likely to get...
A:
Two people between them 4 2 5 960......
Q:
What strategic changes did Target make and what has been the result?
A:
Unlike an industry that was scaling down operations an investment of 7B w......
Q:
Rework Problem 13 assuming the following: In Problem 13, Wildcat, Inc., has estimated sales (in millions) for the next four quarters as follows: Sales for the first quarter of the year after this one...
A:
a With a minimum cash balance of 20 million the short term financial plan will be Below you will find the interest paid or received for each quarter Q......
Q:
4. There is a 9-year bond with an annual coupon rate of 5.25% (paid semi-annually) yielding 6.35% p.a. (continuously compounded). A) Calculate the bond price, duration and convexity. B) Calculate the...
A:
Solution A To calculate the bond price duration and convexity we need to use the bond pricing formula duration formula and convexity formula Let s calculate each one step by step Bond Price The bond p......
Q:
Assume that Speed World uses a periodic inventory system. (See the data given before Problem 8.2A.) Instructions a. Compute the cost of goods sold relating to the sale on July 28 and the ending...
A:
a Cost of goods sold and ending inventory 1 Average cost method Ending inventory at September 30 Ave......
Q:
In physics, it is established that the acceleration due to gravity, g (in m/sec 2 ),at a height h meters above sea level is given by where 6.374 Ã 10 6 is the radius of Earth in meters. (a)...
A:
a b c d Thus the h axis is the horizontal asymptote e To solv......
Q:
In problem, for each polynomial function: (a) List each real zero and its multiplicity. (b) Determine whether the graph crosses or touches the x-axis at each x-intercept. (c) Determine the behavior...
A:
a The real zeros off x x 5 3 x 4 2 are 5 with multiplicity......
Q:
Calculate the internal growth rate and sustainable growth rate for S&S Air. What do these numbers mean?
A:
Click to View...
Q:
Describe the process approach to knowledge management.
A:
The process approach to knowledge management is a systematic and structured method for capturing creating storing sharing and leveraging organizational knowledge to achieve strategic goals and enhance......
Q:
It is said that powerful computers, inference capabilities, and problem-solving heuristics are necessary but not sufficient for solving real problems. Explain.
A:
Solving real world problems often requires more than just powerful computers inference capabilities and problem solving heuristics While these elements are undoubtedly important they are not sufficien......
Q:
What are the major roles of the explanation subsystem?
A:
The explanation subsystem in the context of various systems and applications serves the crucial purpose of providing users with meaningful and informative explanations about system behavior actions or......
Q:
Describe the practice approach to knowledge management.
A:
The practice approach to knowledge management is a perspective that emphasizes the practical application of knowledge within an organization focusing on how knowledge is used in day to day operations......
Q:
Describe the KMS cycle.
A:
The KMS Knowledge Management System cycle is a process that organizations use to effectively capture store retrieve and share knowledge within their workforce It encompasses various stages aimed at ha......
Q:
What is the freezing point of 0.0075 m aqueous calcium chloride, CaCl2? Use the formula of the salt to obtain i.
A:
Begin by noting that i 3 Then c......
Q:
Give codons for the following amino acids: (a) Th (b) Asp (c) Thr
A:
To give the codons for the specified amino acids we need to refer to......
MySQL Cookbook Solutions For Database Developers And Administrators 4th Edition - ISBN: 1492093165 - Free Book