1. Let z,t = 1,...,n, be scalar random variables with Cov(zt, zs) = 0 for ts....

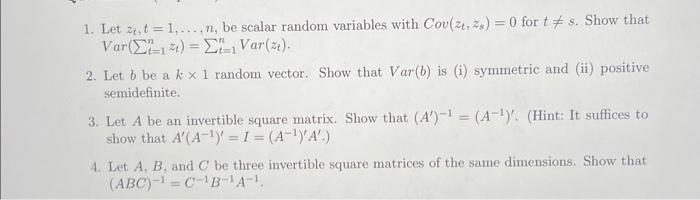
Transcribed Image Text:

1. Let z,t = 1,...,n, be scalar random variables with Cov(zt, zs) = 0 for ts. Show that Var(-1)=E=1 Var(t). 2. Let b be a k x 1 random vector. Show that Var(b) is (i) symmetric and (ii) positive semidefinite. 3. Let A be an invertible square matrix. Show that (A)-1 = (A-¹). (Hint: It suffices to show that A'(A-¹)= I=(A-¹)'A'.) 4. Let A, B, and C be three invertible square matrices of the same dimensions. Show that (ABC)-¹=CB-1A-¹. 1. Let z,t = 1,...,n, be scalar random variables with Cov(zt, zs) = 0 for ts. Show that Var(-1)=E=1 Var(t). 2. Let b be a k x 1 random vector. Show that Var(b) is (i) symmetric and (ii) positive semidefinite. 3. Let A be an invertible square matrix. Show that (A)-1 = (A-¹). (Hint: It suffices to show that A'(A-¹)= I=(A-¹)'A'.) 4. Let A, B, and C be three invertible square matrices of the same dimensions. Show that (ABC)-¹=CB-1A-¹.

Answer rating: 100% (QA)

Answer 22 1 Let 2 t 1n be scaler random v 2 25 0 for tts Cov Cov2 2s 0 2ts are variables with now in... View the full answer