Give an example of two discontinuous functions f and g such that both f + g and f g are continuous. Let T: [0,1] R be a continuous function with T(0) = T(1). Show that there exists ce

  

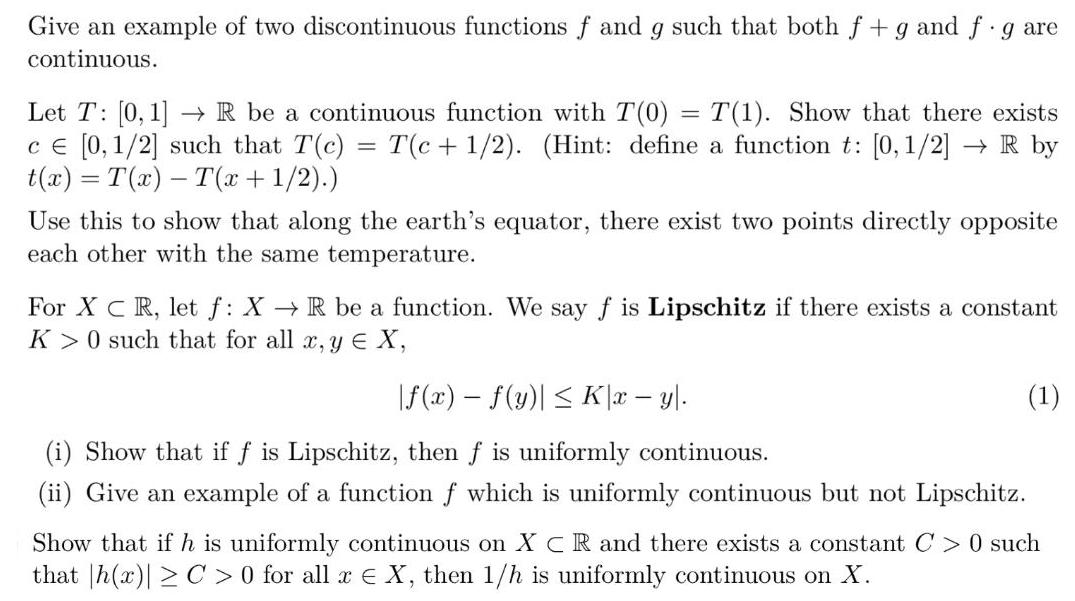
Transcribed Image Text:

Give an example of two discontinuous functions f and g such that both f + g and f g are continuous. Let T: [0,1] → R be a continuous function with T(0) = T(1). Show that there exists ce [0,1/2] such that T(c) T(c + 1/2). (Hint: define a function t: [0,1/2] → R by t(x) = T(x) - T(x + 1/2).) = Use this to show that along the earth's equator, there exist two points directly opposite each other with the same temperature. For XCR, let f: X → R be a function. We say f is Lipschitz if there exists a constant K> 0 such that for all x, y E X, |f(x) = f(y)| ≤ K|x - y\. (i) Show that if f is Lipschitz, then f is uniformly continuous. (ii) Give an example of a function f which is uniformly continuous but not Lipschitz. (1) Show that if h is uniformly continuous on X CR and there exists a constant C> 0 such that h(x) > C> 0 for all x E X, then 1/h is uniformly continuous on X.

• Expert Answer