Use Jacobi's transformation formula to recover Theorem 5.8(i), Problem 5.9 and Theorem 7.10. In particular, for all

Question:

Use Jacobi's transformation formula to recover Theorem 5.8(i), Problem 5.9 and Theorem 7.10. In particular, for all integrable functions \(u: \mathbb{R}^{n} ightarrow[0, \infty)\)

\[
\begin{aligned}
\int u(x+y) \lambda^{n}(d x) & =\int u(x) \lambda^{n}(d x) & & \forall y \in \mathbb{R}^{n}, \\
\int u(t x) \lambda^{n}(d x) & =\frac{1}{t^{n}} \int u(x) \lambda^{n}(d x) & & \forall t>0, \\
\int u(A x) \lambda^{n}(d x) & =\frac{1}{|\operatorname{det} A|} \int u(x) \lambda^{n}(d x) & & \forall A \in \mathbb{R}^{n \times n}, \operatorname{det} A eq 0 .
\end{aligned}
\]

The left-hand side of any of the above equalities exists and is finite if, and only if, the right-hand side exists and is finite.
Why can't we use Theorem 16.4 to prove these formulae?

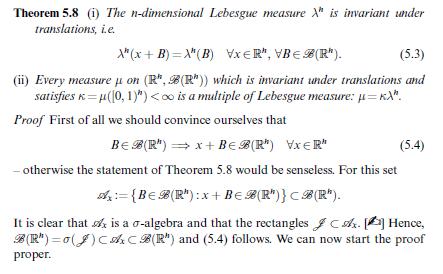
Data from theorem 5.8

Theorem 5.8 (i) The n-dimensional Lebesgue measure X is invariant under translations, i.e. X" (x+B)=X" (B)

Data from problem 5.9

Dilations. Mimic the proof of Theorem 5.8(i) and show that \(t \cdot B:=\{t b: b \in B\}\) is a Borel set for all \(B \in \mathscr{B}\left(\mathbb{R}^{n}ight)\) and \(t>0\). Moreover,
\[
\lambda^{n}(t \cdot B)=t^{n} \lambda^{n}(B) \quad \forall B \in \mathscr{B}\left(\mathbb{R}^{n}ight), \forall t>0
\]

Data from theorem 7.10

Use image measures to give a new proof of Problem 5.9 , i.e. show that
\[
\lambda^{n}(t \cdot B)=t^{n} \lambda^{n}(B) \quad \forall B \in \mathscr{B}\left(\mathbb{R}^{n}ight), \quad \forall t>0
\]