Hansen and Richard (1987) show that an arbitrary gross return (1+R_{i}) can be decomposed as where (R^{*},
Question:
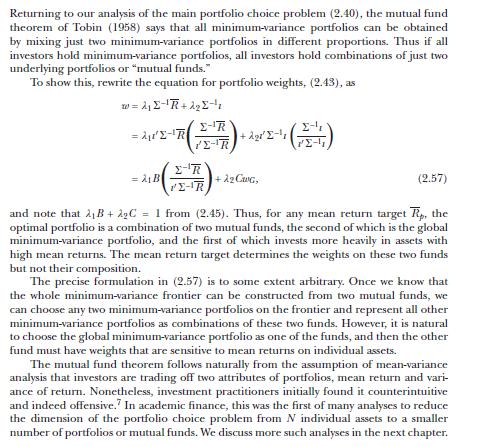
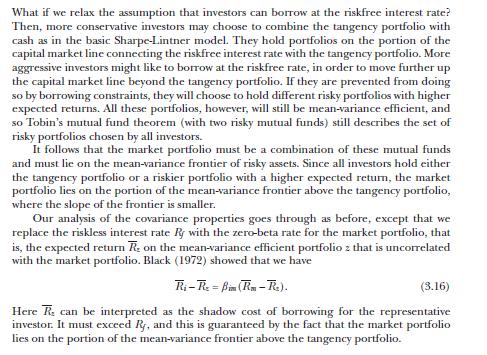
Hansen and Richard (1987) show that an arbitrary gross return \(1+R_{i}\) can be decomposed as
![]()
where \(R^{*}, Z^{*}\), and \(\varepsilon_{i}\) are random variables and \(w_{i}\) is a scalar. This decomposition is orthogonal in the sense that \(\mathrm{E}\left[\left(1+R^{*}ight) Z^{*}ight]=\mathrm{E}\left[\varepsilon_{i}\left(1+R^{*}ight)ight]=\mathrm{E}\left[\varepsilon_{i} Z^{*}ight]=0\). The benchmark return \(1+R^{*} \equiv X^{*} / P\left(X^{*}ight)\) is the gross return on \(X^{*}\), the SDF that is also a payoff, given by (4.35) when the payoff space is finite-dimensional. \(Z^{*} \equiv \operatorname{proj}(1 \mid \underline{Z})\) is the projection of vector 1 (the random variable whose realization equals 1 almost surely) on the space of excess returns, \(\underline{Z}=\{X \in \Xi\) s.t. \(P(X)=0\} .{ }^{16} \varepsilon_{i}\) is a mean-zero residual.
\({ }^{16}\) When the space of excess returns is finite-dimensional, the projection of a variable \(Y\) on the space of excess returns is
![]()
where \(B\) is a column vector of the basis payoffs of \(\underline{Z}\). It is the excess return that best replicates variable \(Y\) in a least-squares sense.
(a) Show that the benchmark return is the gross return with the minimum second (uncentered) moment. Conclude from this result that, in mean-standard deviation space, \(1+R^{*}\) is the return on the mean-variance frontier that is closest to the origin.
(b) Use the definition of \(Z^{*}\) to show that \(Z^{*}\) is the "mean-generating" excess return in the sense that its second (uncentered) moment with any excess return yields the mean of that return, \(\mathrm{E}[Z]=\mathrm{E}\left[Z^{*} Zight]\) for all \(Z \in \underline{Z}\). Then prove that \(Z^{*}\) is the excess return with the maximum Sharpe ratio in absolute value.
(c) Show that the mean-variance frontier is generated by the set of returns that satisfy \(1+R_{i}=1+R^{*}+w_{i} Z^{*}\) for some scalar \(w_{i}\). Use this result to provide an alternative proof of the mutual-fund theorem of section 2.2.5: any mean-variance efficient return can be generated by any two portfolios on the frontier.
(d) Use the decomposition to show that any mean-variance efficient return \(R_{m v}\), except the global minimum-variance return, can serve as the factor in a single-factor beta pricing model of the form
![]()
where \(\mathrm{E}\left[R_{m v, z}ight]\) is the zero-beta rate for \(R_{m v}\), defined in section 3.1.2 (for the case where \(R_{m v}=R_{m}\), the market return).
Data from section 2.2.5
Data from section 3.1.2
Step by Step Answer:

Financial Decisions And Markets A Course In Asset Pricing
ISBN: 9780691160801
1st Edition
Authors: John Y. Campbell





