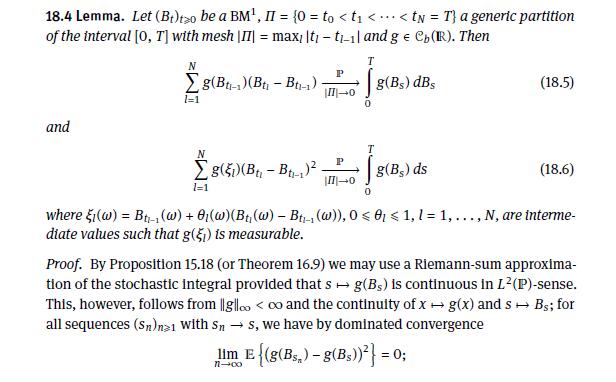
Give a direct proof for the identity \[\begin{aligned}\mathbb{E} & {\left[\left(\sum_{l=1}^{N} g\left(B_{t_{l-1}}ight)\left[\left(B_{t_{l}}-B_{t_{l-1}}ight)^{2}-\left(t_{l}-t_{l-1}ight)ight]ight)^{2}ight] } \\& =\mathbb{E}\left[\sum_{l=1}^{N}\left|g\left(B_{t_{l-1}}ight)ight|^{2}\left[\left(B_{t_{l}}-B_{t_{l-1}}ight)^{2}-\left(t_{l}-t_{l-1}ight)ight]^{2}ight] .\end{aligned}\]

appearing in the proof of Lemma 18.4.

Data From Leema 18.4

18.4 Lemma. Let (Bt)to be a BM, II = {0 to