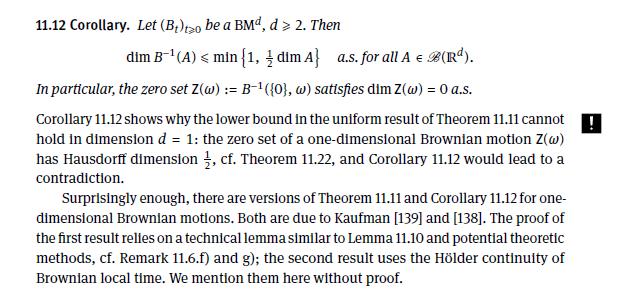
Let (left(B_{t} ight)_{t geqslant 0}) be a one-dimensional Brownian motion. Show that [operatorname{dim} B^{-1}(A) leqslant frac{1}{2}+frac{1}{2} operatorname{dim}

Let \(\left(B_{t}\right)_{t \geqslant 0}\) be a one-dimensional Brownian motion. Show that \[\operatorname{dim} B^{-1}(A) \leqslant \frac{1}{2}+\frac{1}{2} \operatorname{dim} A \quad \text { a.s. for all } A \in \mathscr{B}(\mathbb{R})\]

Apply Corollary 11.12 to \(W_{t}=\left(B_{t}, \beta_{t}\right)\) where \(\beta\) is a further, independent \(\mathrm{BM}^{1}\); observe that \(B^{-1}(A)=W^{-1}(A \times \mathbb{R})\).

Data From  Corollary 11.12

Transcribed Image Text:

11.12 Corollary. Let (Bt)to be a BMd, d> 2. Then dim B(A)