Suppose a trial can result in precisely one of k mutually exclusive eventsA 1 ,
Question:
Suppose a trial can result in precisely one of k mutually exclusive events A1, · · ·,Ak with probabilities p1, · · ·, pk, respectively, where P1 + · · · + Pk = 1. Suppose that n independent trials are performed. Show that the probability of getting x1A1’s, · · ·, xkAk's is
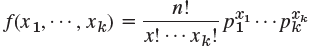
where 0 ≤ xj ≤ n, j = 1, · · ·,k, and x1 + · · · + xk = n. The distribution having this probability function is called the multinomial distribution.
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
We can approach this problem using the following reasoning Consider a sequence of n independent tria...View the full answer
Answered By
Brian Otieno
I'm Brian , an experienced professional freelancer with countless hours of success in freelancing many subjects in different disciplines. Specifically, I have handled many subjects and excelled in many disciplines. I have worked on many Computer Science projects and have been able to achieve a lot in that field. Additionally, I have handled other disciplines like History, Humanities, Social Sciences, Political science, Health care and life science, and Religion / Theology. My experience generally in these subjects has made me able to deliver high-quality projects in a very timely fashion. I am very reliable at my job and will get the work done in time, no matter what. In Addition, I have managed to ensure that the work meets my client's expectations and does not cause an error. I am a hard-working and diligent person who is highly responsible for everything I do. Generally, Freelancing has made me more accountable for doing my job. Additionally, I have had a passion for writing for the last seven years in this field.
0 Reviews
10+ Question Solved
Related Book For 
Question Posted:
