(i) Show that (lambda^{1}((a, b))=b-a) for all (a, b in mathbb{R}, a leqslant b). [ approximate ((a,...
Question:
(i) Show that \(\lambda^{1}((a, b))=b-a\) for all \(a, b \in \mathbb{R}, a \leqslant b\).
[ approximate \((a, b)\) by half-open intervals and use Proposition 4.3 (vi), (vii).]
(ii) Let \(H \subset \mathbb{R}^{2}\) be a hyperplane which is perpendicular to the \(x_{1}\)-direction (that is to say, \(H\) is a translate of the \(x_{2}\)-axis). Show that \(H \in \mathscr{B}\left(\mathbb{R}^{2}ight)\) and \(\lambda^{2}(H)=0\).
[ note that \(H \subset y+\cup_{k \in \mathbb{N}} A_{k}\) for some \(y\) and \(A_{k}=\left[-\epsilon 2^{-k}, \epsilon 2^{-k}ight) \times[-k, k)\).]
(iii) State and prove the \(\mathbb{R}^{n}\)-analogues of parts (i) and (ii).
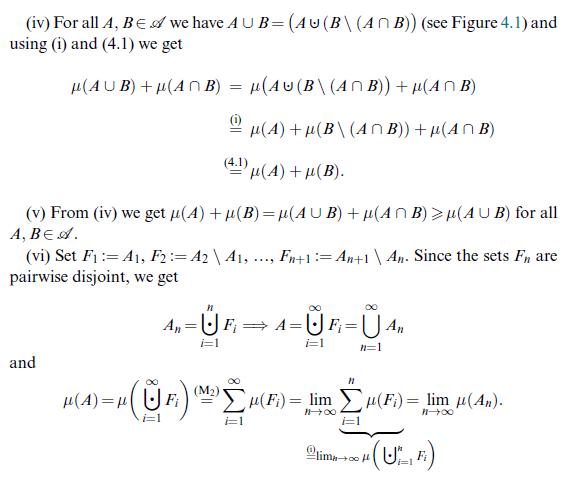
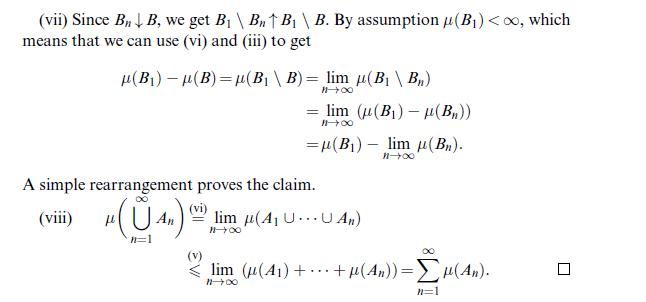
Data from proposition 4.3
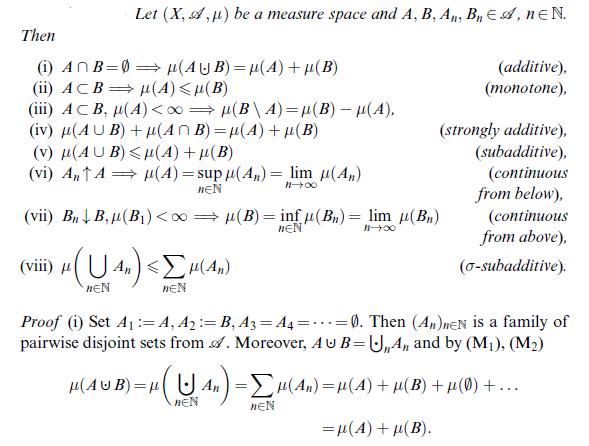
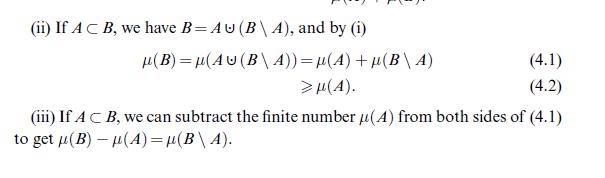
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





