Let \((X, \mathscr{A}, \mu)\) be a measure space. Show the following variant of Theorem 9.6. If \(u_{n} \geqslant 0\) are measurable functions such that for some \(u\) we have
\[
\exists K \in \mathbb{N} \quad \forall x: u_{n+K}(x) \uparrow u(x) \text { as } n ightarrow \infty
\]
then \(u \geqslant 0\) is measurable and \(\int u_{n} d \mu \uparrow \int u d \mu\).
Show that we cannot replace the above condition with
\[
\forall x \quad \exists K \in \mathbb{N}: u_{n+K}(x) \uparrow u(x) \text { as } n ightarrow \infty
\]

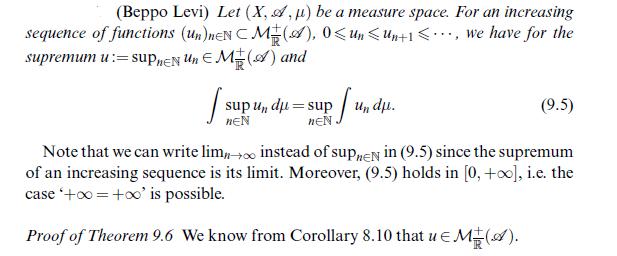
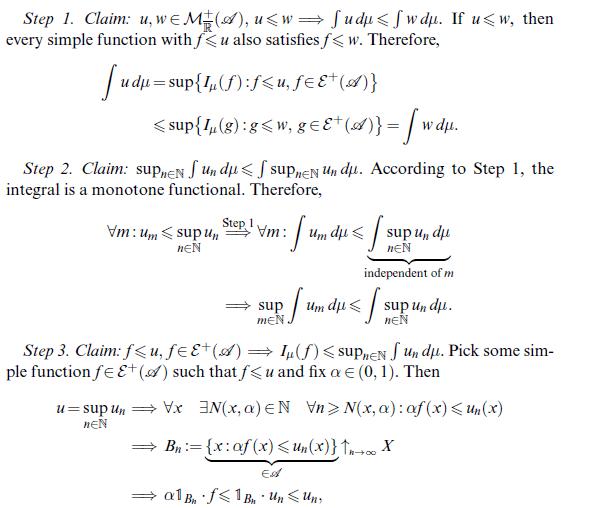
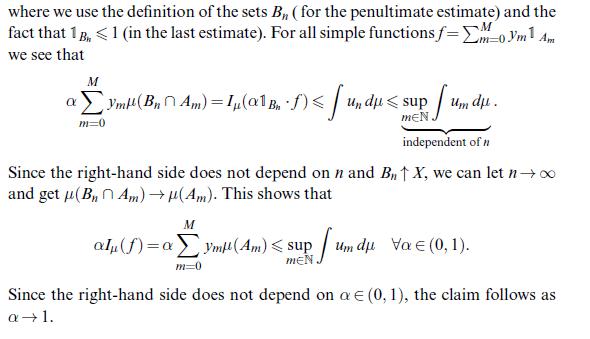
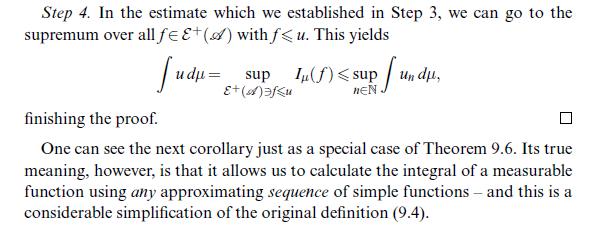
Data from theorem 9.6

Transcribed Image Text:

(Beppo Levi) Let (X, A, ) be a measure space. For an increasing sequence of functions (Un)neN CM(A), 0un < Un+1