Let (left(mathcal{C}_{(mathrm{o})}, mathscr{B}left(mathcal{C}_{(mathrm{o})} ight), mu ight)) be the canonical Wiener space (we assume (d=1) ). We will

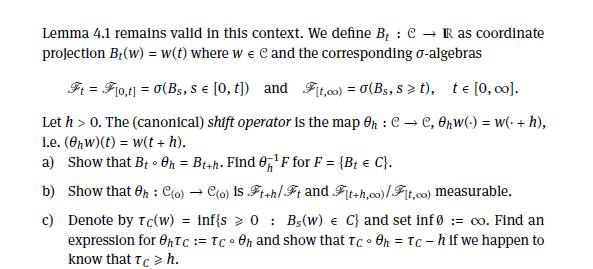
Let \(\left(\mathcal{C}_{(\mathrm{o})}, \mathscr{B}\left(\mathcal{C}_{(\mathrm{o})}\right), \mu\right)\) be the canonical Wiener space (we assume \(d=1\) ). We will now consider the space \(\mathcal{C}\), again equipped with the metric of locally uniform convergence and the Borel \(\sigma\)-algebra \(\mathscr{B}(\mathcal{C})\). It is straightforward to check that Lemma 4.1 remains valid in this context. We define \(B_{t}: \mathcal{C} \rightarrow \mathbb{R}\) as coordinate projection \(B_{t}(w)=w(t)\) where \(w \in \mathcal{C}\) and the corresponding \(\sigma\)-algebras

\[\mathscr{F}_{t}=\mathscr{F}_{[0, t]}=\sigma\left(B_{s}, s \in[0, t]\right) \quad \text { and } \quad \mathscr{F}_{[t, \infty)}=\sigma\left(B_{s}, s \geqslant t\right), \quad t \in[0, \infty] .\]

Let \(h>0\). The (canonical) shift operator is the map \(\theta_{h}: \mathcal{C} \rightarrow \mathcal{C}, \theta_{h} w(\cdot)=w(\cdot+h)\), i.e. \(\left(\theta_{h} w\right)(t)=w(t+h)\).

a) Show that \(B_{t} \circ \theta_{h}=B_{t+h}\). Find \(\theta_{h}^{-1} F\) for \(F=\left\{B_{t} \in C\right\}\).

b) Show that \(\theta_{h}: \mathcal{C}_{(0)} \rightarrow \mathcal{C}_{(0)}\) is \(\mathscr{F}_{t+h} / \mathscr{F}_{t}\) and \(\mathscr{F}_{[t+h, \infty)} / \mathscr{F}_{[t, \infty)}\) measurable.

c) Denote by \(\tau_{C}(w)=\inf \left\{s \geqslant 0: B_{S}(w) \in C\right\}\) and set \(\inf \emptyset:=\infty\). Find an expression for \(\theta_{h} \tau_{C}:=\tau_{C} \circ \theta_{h}\) and show that \(\tau_{C} \circ \theta_{h}=\tau_{C}-h\) if we happen to know that \(\tau_{C} \geqslant h\).

Data From  Lemma 4.1

Transcribed Image Text:

Lemma 4.1 remains valid in this context. We define Bt e R as coordinate projection B(w) = w(t) where we C and the corresponding -algebras F = F[0,t] =(Bs, S [0, t]) and Ft,00) = 0(Bs, St), te [0, 0]. Let h>0. The (canonical) shift operator is the map hee, Ohw() = w(+ h), 1.e. (hw)(t) = w(t + h). a) Show that Bt Oh = Bt+h. Find F for F = {B = C}. b) Show that Oh: C(o) c) Denote by Tc(w) = t C(o) Is Ft+hl Ft and Ft+h,co)/Ft,co) measurable. = inf{s 0 Bs(w) = C) and set inf0 = co. Find an expression for htc := Tc Oh and show that Tc Oh Tc - h if we happen to know that Tch.