solve the circled questions 31. Ax+By C; for y (B 0) 32. y = mx + b;

solve the circled questions

Transcribed Image Text:

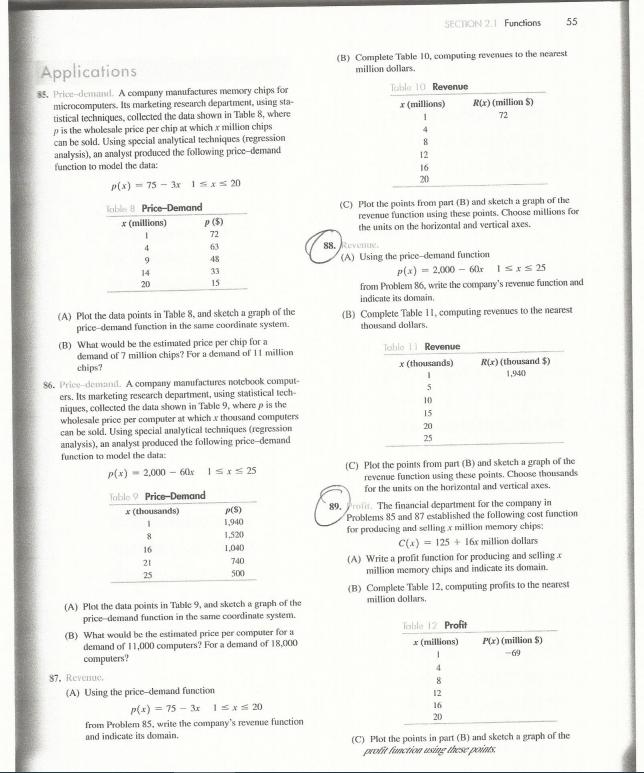
31. Ax+By C; for y (B 0) 32. y = mx + b; for m 34. C (F-32); for F Solve Problems 35 and 36 and graph. 35.-34-7x < 18 36.-1083μ-6 37. What can be said about the signs of the numbers a and b in each case? (A) ab > 0 ght 38. What can be said about the signs of the numbers a, b, and c in each case? (A) abc > () (C) 20 33. FC + 32; for C >0 (B) ab < 0 (B) (D) ab <0 <0 be be 39. If both a and bare positive numbers and bla is greater than 1. then is a - b positive or negative? 40. If both a and bare negative numbers and bla is greater than 1, then is a - b positive or negative? In Problems 41-46, discuss the validity of each statement. If the statement is true, explain why. If not, give a counterexample. 41. If the intersection of two open intervals is nonempty, then their intersection is an open interval. 42. If the intersection of two closed intervals is nonempty, then their intersection is a closed interval. 43. The union of any two open intervals is an open interval. 44. The union of any two closed intervals is a closed interval. 45. If the intersection of two open intervals is nonempty, then their union is an open interval. 46. If the intersection of two closed intervals is nonempty, then their union is a closed interval. Applications 47. Ticket sales. A rock concert brought in $432,500 on the sale of 9,500 tickets. If the tickets sold for $35 and $55 each, how many of each type of ticket were sold? 48. Parking meter coins. An all-day parking meter takes only dimes and quarters. If it contains 100 coins with a total value of $14.50, how many of each type of coin are in the meter? SECTION 1.1 Linear Equations and Inequalities 11 50. IRA. Refer to Problem 49. How should you divide your money between Fund A and Fund B to produce an annual in- terest income of $30,000? 51. Car prices. If the price change of cars parallels the change in the CPI (see Table 2 in Example 10), what would a car sell for (to the nearest dollar) in 2012 if a comparable model sold for $10,000 in 1999? 52. Home values. If the price change in houses parallels the CPI (see Table 2 in Example 10), what would a house valued at $200,000 in 2012 be valued at (to the nearest dollar) in 1960? 53. Retail and wholesale prices. Retail prices in a department store are obtained by marking up the wholesale price by 40%. That is, the retail price is obtained by adding 40% of the wholesale price to the wholesale price. (A) What is the retail price of a suit if the wholesale price is $300? (B) What is the wholesale price of a pair of jeans if the retail price is $77? 54. Retail and sale prices. Sale prices in a department store are obtained by marking down the retail price by 15%. That is, the sale price is obtained by subtracting 15% of the retail price from the retail price. (A) What is the sale price of a hat that has a retail price of $60? (B) What is the retail price of a dress that has a sale price of $136? 55. Equipment rental. A golf course charges $52 for a round of golf using a set of their clubs, and S44 if you have your own clubs. If you buy a set of clubs for $270, how many rounds must you play to recover the cost of the clubs? 56. Equipment rental. The local supermarket rents carpet cleaners for $20 a day. These cleaners use shampoo in a special cartridge that sells for $16 and is available only from the supermarket. A home carpet cleaner can be purchased for $300. Shampoo for the home cleaner is readily available for $9 a bottle. Past experience has shown that it takes two shampoo cartridges to clean the 10-foot-by-12-foot carpet in your living room with the rented cleaner. Cleaning the same area with the home cleaner will consume three bottles of shampoo. If you buy the home cleaner, how many times must you clean the living-room carpet to make buying cheaper than renting? 57. Sales commissions. One employee of a computer store is paid a base salary of $2.000 a month plus an 8% commission on all sales over $7,000 during the month. How much must the employee sell in one month to earn a total of $4,000 for the month? 58. Sales commissions. A second employee of the computer store in Problem 57 is paid a base salary of $3,000 a month plus a 5% commission on all sales during the month. 49. IRA. You have $500,000 in an IRA (Individual Retirement Account) at the time you retire. You have the option of investing this money in two funds: Fund A pays 5.2% annually and Fund B pays 7.7% annually. How should you divide your money be- tween Fund A and Fund B to produce an annual interest income of $34,000? Printed by Coretta Frazier (coratafiazier@att.nation 10/25/2015 from 107,203 129 130 authorized to use until 12/12/2015 Use beyond the authorized user or valid subscription date represents a copyright violation (A) How much must this employee sell in one month to earn a total of $4,000 for the month? (B) Determine the sales level at which both employees receive the same monthly income. 31. Ax+By C; for y (B 0) 32. y = mx + b; for m 34. C (F-32); for F Solve Problems 35 and 36 and graph. 35.-34-7x < 18 36.-1083μ-6 37. What can be said about the signs of the numbers a and b in each case? (A) ab > 0 ght 38. What can be said about the signs of the numbers a, b, and c in each case? (A) abc > () (C) 20 33. FC + 32; for C >0 (B) ab < 0 (B) (D) ab <0 <0 be be 39. If both a and bare positive numbers and bla is greater than 1. then is a - b positive or negative? 40. If both a and bare negative numbers and bla is greater than 1, then is a - b positive or negative? In Problems 41-46, discuss the validity of each statement. If the statement is true, explain why. If not, give a counterexample. 41. If the intersection of two open intervals is nonempty, then their intersection is an open interval. 42. If the intersection of two closed intervals is nonempty, then their intersection is a closed interval. 43. The union of any two open intervals is an open interval. 44. The union of any two closed intervals is a closed interval. 45. If the intersection of two open intervals is nonempty, then their union is an open interval. 46. If the intersection of two closed intervals is nonempty, then their union is a closed interval. Applications 47. Ticket sales. A rock concert brought in $432,500 on the sale of 9,500 tickets. If the tickets sold for $35 and $55 each, how many of each type of ticket were sold? 48. Parking meter coins. An all-day parking meter takes only dimes and quarters. If it contains 100 coins with a total value of $14.50, how many of each type of coin are in the meter? SECTION 1.1 Linear Equations and Inequalities 11 50. IRA. Refer to Problem 49. How should you divide your money between Fund A and Fund B to produce an annual in- terest income of $30,000? 51. Car prices. If the price change of cars parallels the change in the CPI (see Table 2 in Example 10), what would a car sell for (to the nearest dollar) in 2012 if a comparable model sold for $10,000 in 1999? 52. Home values. If the price change in houses parallels the CPI (see Table 2 in Example 10), what would a house valued at $200,000 in 2012 be valued at (to the nearest dollar) in 1960? 53. Retail and wholesale prices. Retail prices in a department store are obtained by marking up the wholesale price by 40%. That is, the retail price is obtained by adding 40% of the wholesale price to the wholesale price. (A) What is the retail price of a suit if the wholesale price is $300? (B) What is the wholesale price of a pair of jeans if the retail price is $77? 54. Retail and sale prices. Sale prices in a department store are obtained by marking down the retail price by 15%. That is, the sale price is obtained by subtracting 15% of the retail price from the retail price. (A) What is the sale price of a hat that has a retail price of $60? (B) What is the retail price of a dress that has a sale price of $136? 55. Equipment rental. A golf course charges $52 for a round of golf using a set of their clubs, and S44 if you have your own clubs. If you buy a set of clubs for $270, how many rounds must you play to recover the cost of the clubs? 56. Equipment rental. The local supermarket rents carpet cleaners for $20 a day. These cleaners use shampoo in a special cartridge that sells for $16 and is available only from the supermarket. A home carpet cleaner can be purchased for $300. Shampoo for the home cleaner is readily available for $9 a bottle. Past experience has shown that it takes two shampoo cartridges to clean the 10-foot-by-12-foot carpet in your living room with the rented cleaner. Cleaning the same area with the home cleaner will consume three bottles of shampoo. If you buy the home cleaner, how many times must you clean the living-room carpet to make buying cheaper than renting? 57. Sales commissions. One employee of a computer store is paid a base salary of $2.000 a month plus an 8% commission on all sales over $7,000 during the month. How much must the employee sell in one month to earn a total of $4,000 for the month? 58. Sales commissions. A second employee of the computer store in Problem 57 is paid a base salary of $3,000 a month plus a 5% commission on all sales during the month. 49. IRA. You have $500,000 in an IRA (Individual Retirement Account) at the time you retire. You have the option of investing this money in two funds: Fund A pays 5.2% annually and Fund B pays 7.7% annually. How should you divide your money be- tween Fund A and Fund B to produce an annual interest income of $34,000? Printed by Coretta Frazier (coratafiazier@att.nation 10/25/2015 from 107,203 129 130 authorized to use until 12/12/2015 Use beyond the authorized user or valid subscription date represents a copyright violation (A) How much must this employee sell in one month to earn a total of $4,000 for the month? (B) Determine the sales level at which both employees receive the same monthly income.

Answer rating: 100% (QA)

Solution Find the CPI ratio We need to know the CPI values for both years Lets assume the CPI in 201... View the full answer