Show that (a) Show that E(X - ) = 0, 2 = E(X 2 ) - 2 . (b) Prove (10)-(12). (c) Find all the moments of the uniform distribution on an interval x b. (d) The skewness of a random variable X is defined by Show that for a symmetric distribution (whose third
Chapter 24, PROBLEM SET 24.6 #20
Show that
(a) Show that E(X - μ) = 0, σ2 = E(X2) - μ2.
(b) Prove (10)-(12).
(c) Find all the moments of the uniform distribution on an interval α ≤ x ≤ b.
(d) The skewness ϒ of a random variable X is defined by
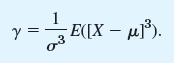
Show that for a symmetric distribution (whose third central moment exists) the skewness is zero.
(e) Find the skewness of the distribution with density f(x) = xe-x when x > 0 and f(x) = 0 otherwise. Sketch f(x).
(f) Find a nonsymmetric discrete distribution with 3 possible values, mean 0, and skewness 0.
DistributionThe word "distribution" has several meanings in the financial world, most of them pertaining to the payment of assets from a fund, account, or individual security to an investor or beneficiary. Retirement account distributions are among the most...
This problem has been solved!
Do you need an answer to a question different from the above? Ask your question!
Related Book For 
