The first term in Equation 11.32 comes from the e it /2 part of Cos(t), and the
Question:
The first term in Equation 11.32 comes from the eiωt/2 part of Cos(ωt), and the second from e-iωt. Thus dropping the first term is formally equivalent to writing Ĥ' = (V/2)e-iωt, which is to say,

(The latter is required to make the Hamiltonian matrix hermitian—or, if you prefer, to pick out the dominant term in the formula analogous to Equation 11.32 for Ca(t).) Rabi noticed that if you make this so-called rotating wave approximation at the beginning of the calculation, Equation 11.17 can be solved exactly, with no need for perturbation theory, and no assumption about the strength of the field.
(a) Solve Equation 11.17 in the rotating wave approximation (Equation 11.36), for the usual initial conditions: Ca(0) = 1, Cb(0) = 0. Express your results (Ca(t) and Cb(t) in terms of the Rabi flopping frequency,
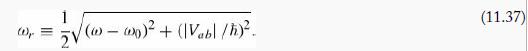
(b) Determine the transition probability, Pa→b(t), and show that it never exceeds 1. Confirm that |Ca(t) |2 + |Cb(t) |2 = 1.
(c) Check that Pa→b(t) reduces to the perturbation theory result (Equation 11.35) when the perturbation is “small,” and state precisely what small means in this context, as a constraint on V.
(d) At what time does the system first return to its initial state?

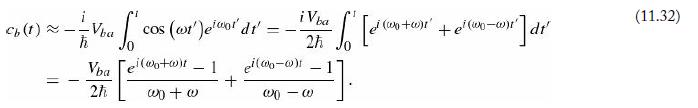

Step by Step Answer:

Introduction To Quantum Mechanics
ISBN: 9781107189638
3rd Edition
Authors: David J. Griffiths, Darrell F. Schroeter





