Extend Plancherel's theorem (Theorem 19.20 ) to show that [int widehat{u}(xi) overline{widehat{v}(xi)} d xi=(2 pi)^{-n} int u(x)
Question:
Extend Plancherel's theorem (Theorem 19.20 ) to show that
\[\int \widehat{u}(\xi) \overline{\widehat{v}(\xi)} d \xi=(2 \pi)^{-n} \int u(x) \overline{v(x)} d x \quad \forall u, v \in L_{\mathbb{C}}^{2}\left(\lambda^{n}ight)\]
Data from theorem 19.20
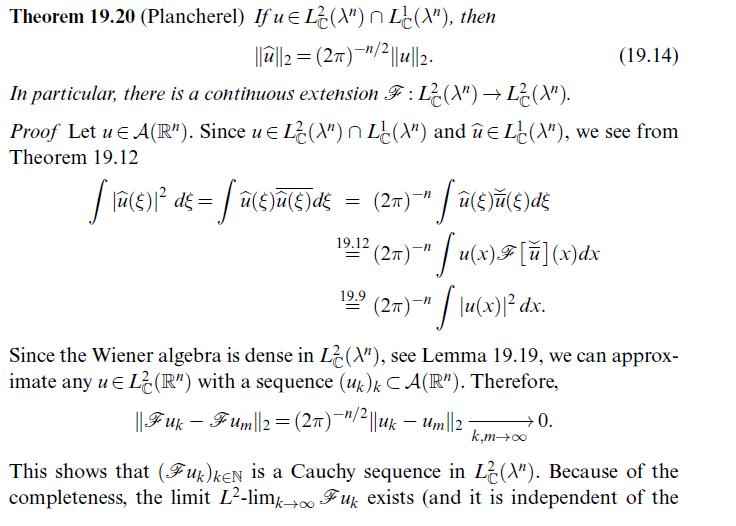
![approximating sequence [2]!) and defines an element Fu= L(X"); the identity (19.14) remains valid since the](https://dsd5zvtm8ll6.cloudfront.net/images/question_images/1705/9/0/5/58565ae0db1a07bd1705905580843.jpg)
Transcribed Image Text:
Theorem 19.20 (Plancherel) If u = L(X") L(X"), then ||||2 = (2)-/2||u|| 2. In particular, there is a continuous extension F: L(X") L(X"). Proof Let u A(R"). Since u = L(X") L(X") and = L(X"), we see from Theorem 19.12 [ \(&) d = [(E)(E)d = 19.9 (2)" [()(E)d (27)" [u(x) F[] (x) dx (27)" [|u(x)| dx. Since the Wiener algebra is dense in L(X"), see Lemma 19.19, we can approx- imate any u L(R") with a sequence (uk) k CA(R"). Therefore, ||FUK - FUm||2 = (2)-n/2 ||uk Um||2 - - (19.14) 0. k,mo This shows that (Fuk) kEN is a Cauchy sequence in L(X"). Because of the completeness, the limit L-lim-o Fuk exists (and it is independent of the
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Answer rating: 100% (1 review)
and so setting u U and so setting IU Observe that for complex n...View the full answer

Answered By

Joram mutua
I am that writer who gives his best for my student/client. Anything i do, i give my best. I have tutored for the last five years and non of my student has ever failed, they all come back thanking me for the best grades. I have a degree in economics, but i have written academic papers for various disciplines due to top-notch research Skills.In additional, I am a professional copywriter and proofreader.
5.00+
2+ Reviews
10+ Question Solved
Related Book For 

Question Posted:
Students also viewed these Business questions
-
The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-6. On December 12, Irene purchased the building where her store is located. She paid...
-
The following additional information is available for the Dr. Ivan and Irene Incisor family from Chapters 1-5. Ivan's grandfather died and left a portfolio of municipal bonds. In 2012, they pay Ivan...
-
Emily Jackson (Social Security number 765-12-4326) and James Stewart (Social Security number 466-74-9932) are partners in a partnership that owns and operates a barber shop. The partnership's first...
-
A local university is considering changes to its class structure in an effort to increase professor productivity. The old schedule had each professor teaching 5 classes per week, with each class...
-
A cable passes around three 30-mm-radius pulleys and supports two blocks as shown. Pulleys C and E are locked to prevent rotation, and the coefficients of friction between the cable and the pulleys...
-
If F cv = 2.337, draw a sampling distribution of F, label the rare and common zones, locate F = 5.66, and determine, for this F value, if the null hypothesis should be rejected.
-
On April 4, 2014, Athanasios Valsamis lost his appeal to get his money back from a friend to whom he had loaned \($700,000.\) As you will read, this case underscores the consequence of failing to...
-
Jerry Karron, an auditor with Joshi CPAs, is performing a review of Duncan Companys Inventory account. Duncan did not have a good year, and top management is under pressure to boost reported income....
-
NTP Inc. raises its first venture round of 7,000,000.00 at a pre-money valuation of 14,000,000.00 from A-Capital. Before the round, the company had 100,000.00 shares outstanding, solely distributed...
-
Let \(\mu\) be a finite measure on \(\left(\mathbb{R}^{n}, \mathscr{B}\left(\mathbb{R}^{n}ight)ight)\) and set \(\chi(\xi):=\widehat{\mu}(\xi)\). Show that \(\chi\) is real-valued if, and only if,...
-
Calculate the Fourier transform of the following functions/measures on \(\mathbb{R}\) : (a) \(\mathbb{1}_{[-1,1]}(x)\), (b) \(\mathbb{1}_{[-1,1]} \star \mathbb{1}_{[-1,1]}(x)\), (c) \(e^{-x}...
-
Which of the following equations represents a circle in the xy-plane that intersects the x-axis at (3, 0) and (9, 0)? A) (x-6) + (y-4) = 25 B) (x-3)2+(y - 9) = 25 C) (x-6) + (y-4) = 36 (x-3) + (y -...
-
If you want to buy industrial die-cutting machinery within 4 years with an estimated value of $525,000, How much should you save in the bank annually if it offers you an annual interest of 8%?
-
You work as a drug and alcohol support worker. Kane has been referred to your service for 10 sessions as part of his Community Corrections Order. Kane is explaining his relationship with Mahlee and...
-
What is the time-weighted rate of return of the first quarter? Date 31 Dec 31 Jan 12 Feb 17 Feb 28 Feb 28 Mar 31 Mar Fair portfolio value 300 314 250 277 260 270 290 End of day ECF 30 -20 10
-
A double-entry accounting system that is in balance has which characteristics?
-
This Youtube video shows you how graph databases are applied to fraud detection https://www.youtube.com/watch?v=7By0hsUj3nU Form groups and answer the following Q1. List some typical types of fraud....
-
During the first month of operations ended July 31, 2010, Tri-State Bakers Inc. baked 7,000 cakes, of which 6,400 were sold. Operating data for the month are summarized as follows: During August,...
-
Why should you not model a decision variable as a random variable with a probability distribution?
-
Let \(\left(B_{t}ight)_{t \geqslant 0}\) be a \(\mathrm{BM}^{1}\). Find an SDE which has \(X_{t}=t B_{t}, t \geqslant 0\), as unique solution.
-
Let \(\left(B_{t}ight)_{t \geqslant 0}\) be a \(\mathrm{BM}^{1}\). The solution of the \(\mathrm{SDE}\) \[d X_{t}=-\beta X_{t} d t+\sigma d B_{t}, \quad X_{0}=\xi\] with \(\beta>0\) and \(\sigma \in...
-
Let \((B(t))_{t \in[0,1]}\) be a \({B M^{1}}^{1}\) and set \(t_{k}=k / 2^{n}\) for \(k=0,1, \ldots, 2^{n}\) and \(\Delta t=2^{-n}\). Consider the difference equation \[\Delta...
-
Duane, a licensed life insurance agent with many years of experience with high net worth individuals, meets a referral for the first time. The referral, named Darya, owns a retail exporting company....
-
For both scenarios what type of ppe could be useful in deterring temperature extremes and what theories of any could be applied to an accident or incident involving temperature extremes? 1. Put...
-
Trouble on a coding project that involves changing C code into MIPS assembly language. Here's the C code I need to convert. int n; cout < < "Input number --> cin >>n; int a == 1, sum=0; do { sum +=...

Study smarter with the SolutionInn App


