Stielties measure (3). Let \((\mathbb{R}, \mathscr{B}(\mathbb{R}), \mu)\) be a finite measure space and denote by \(F\) the left-continuous distribution function of \(\mu\) as in Problem 6.1. Use Lebesgue's decomposition theorem (Theorem 20.4 ) to show that we can decompose \(F=F_{1}+F_{2}+F_{3}\) and, accordingly, \(\mu=\mu_{1}+\mu_{2}+\mu_{3}\) in such a way that

(a) \(F_{1}\) is discrete, i.e. \(\mu_{1}\) is the countable sum of weighted Dirac \(\delta\)-measures;

(b) \(F_{2}\) is absolutely continuous (see Problem 20.6 );

(c) \(F_{3}\) is continuous and singular, i.e. \(\mu_{3} \perp \lambda^{1}\).

Data from theorem 20.4

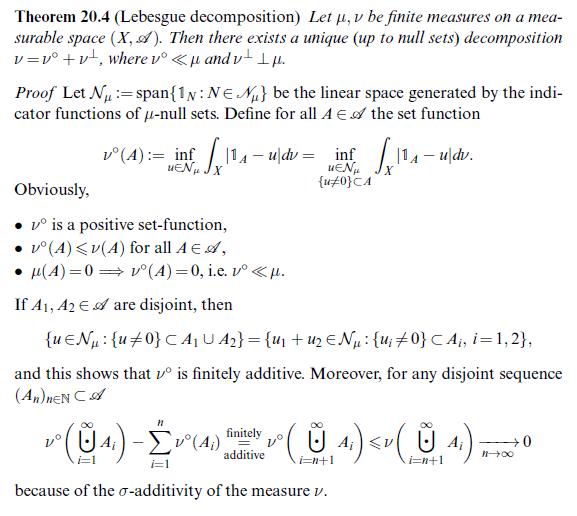
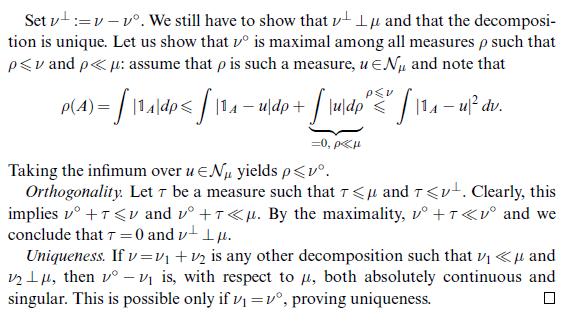
Data from problem 20.6

Bounded variation and absolute continuity. Let \(\lambda\) be one-dimensional Lebesgue measure.
A function \(F:[a, b] ightarrow \mathbb{R}\) is said to be
absolutely continuous (AC) if for each \(\epsilon>0\) there is a \(\delta>0\) such that \(\sum_{n=1}^{N}\left|F\left(y_{n}ight)-F\left(x_{n}ight)ight| \leqslant \epsilon \quad\) for \(\quad\) all \(\quad a \leqslant x_{1}\(\sup \sum_{n=1}^{N}\left|F\left(t_{n}ight)-F\left(t_{n-1}ight)ight|\) is finite, where the sup extends over all finite partitions \(t_{0}=a

Transcribed Image Text:

Theorem 20.4 (Lebesgue decomposition) Let u, v be finite measures on a mea- surable space (X, A). Then there exists a unique (up to null sets) decomposition v=v +v+, where v