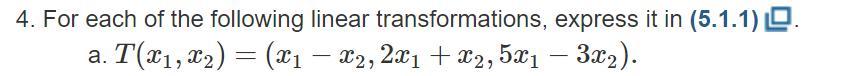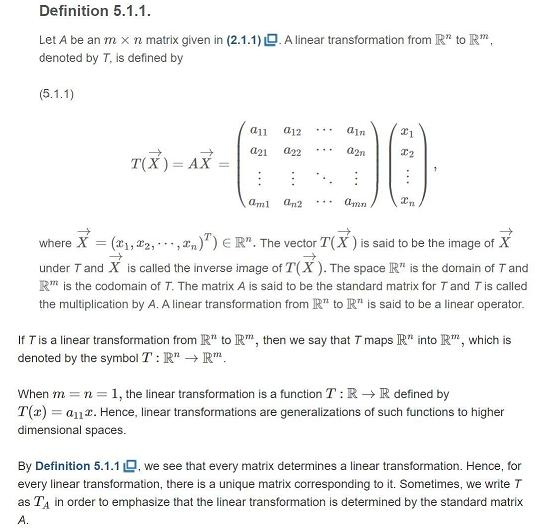
4. For each of the following linear transformations, express it in (5.1.1) O. a. T(x1, x2) = (x1 x2, 2x1 +x2, 5x1 3x2). Definition 5.1.1. Let A be an m x n matrix given in (2.1.1)O. A linear transformation from R" to R". denoted by T, is defined by (5.1.1) a11 a12 din ... a21 a22 a2n ... T(X)= AX = %3D Aml amn In ... where X = (*1, L2,- ,n)") E R". The vector T(X ) is said to be the image of X under Tand X is called the inverse image of T(X ). The space R" is the domain of Tand R" is the codomain of T. The matrix A is said to be the standard matrix for Tand Tis called the multiplication by A. A linear transformation from R" to R" is said to be a linear operator. If Tis a linear transformation from R" to R", then we say that T maps R" into R", which is denoted by the symbol T: R" R" When m =n = 1, the linear transformation is a function T:R -R defined by T(x) = a1r. Hence, linear transformations are generalizations of such functions to higher dimensional spaces. By Definition 5.1.1 0, we see that every matrix determines a linear transformation. Hence, for every linear transformation, there is a unique matrix corresponding to it. Sometimes, we write T as T, in order to emphasize that the linear transformation is determined by the standard matrix A.