Question: Let f be the function defined by f(x) = ?ln(x) for 0 1. This question does not permit the use of a calculator. Determine whether

Let f be the function defined by f(x) = ?ln(x) for 0

1. This question does not permit the use of a calculator. Determine whether region R has a finite area. If so, find the area. If not, explain why.

2. This question does not permit the use of a calculator. Determine whether the solid generated by revolving region R about the y-axis has a finite volume. If so, find the volume. If not, explain why.

3. The formula for the arc length from a to b of a differentiable function is

L = b a 1 + [f'(x)] dx

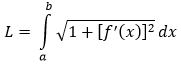
Find the length of the curve f(x) from x = 0.5 to x = 1. Your work must show the integral, but you may use your calculator to evaluate it. Give 3 decimal places in your answer.

4. g(x) = x ². Find the area bounded by f(x), g(x) and the x-axis in the interval [0.5, 1]. Your work must show the integral, but you may use your calculator to evaluate it. Give 3 decimal places in your final answer.

L = b a 1 + [f'(x)] dx

Step by Step Solution

★★★★★

3.45 Rating (161 Votes )

There are 3 Steps involved in it

1 Expert Approved Answer

Step: 1 Unlock

To solve these problems lets tackle each one step by step 1 Determine whether region R has a finite area Given fx x lnx lets focus on the interval 0 x ... View full answer

Question Has Been Solved by an Expert!

Get step-by-step solutions from verified subject matter experts

Step: 2 Unlock

Step: 3 Unlock

Students Have Also Explored These Related Mathematics Questions!

Let f be the function whose graph is given. (a) Estimate the value of f (2). (b) Estimate the values of x such that f(x) = 3. (c) State the domain of f. (d) State the range of f. (e) On what interval...

Let f be the function whose graph is given. (a) Estimate the value of f(2). (b) Estimate the values of such that f(x) = 3. (c) State the domain of f. (d) State the range of f. (e) On what interval is...

Let F be the function such that F(n) is the sum of the first n positive integers. Give a recursive definition of F(n).

Find the volume of the solid generated by revolving the given region bounded by the line and curve about the x-axis. 8 The region between the curve y - and the x-axis from x = 12 to x = 18. The...

1. 3 2 Consider the function f(x) = x - 3x - 9x + 10, x (a) . Find the equation of the straight line passing through the maximum and minimum points of the graph y = f(x). (4) (b) Show that the point...

In a Hopfield neural network configured as an associative memory, with all of its weights trained and fixed, what three possible behaviours may occur over time in configuration space as the net...

NAME: SCORE: 250 Points (An answer, even if "correct" but lacking work, will NOT receive full credit!) 1. Evaluate each integral by making an appropriate substitution. (a) X dx + 4 flex - ex (b ) Joe...

Let A, B be sets. Define: (a) the Cartesian product (A B) (b) the set of relations R between A and B (c) the identity relation A on the set A [3 marks] Suppose S, T are relations between A and B, and...

list Evaluate the following integral K dx 2 + 4x + 20 Question 1 Question 2 dx 2 + 4X + 20 tan * + C (Type an exact answer.) O Question 3 O Question 4 O Question 5 Question 6ng thuc chinh... (14)...

Set Student Name: 1. Answer true or false for each part, and if false, explain your answer. a. The point estimate for the population mean, , of an x distribution is x-bar, computed from a random...

1. Acquisition of Shares On June 1 Eastern Corporation acquired 75,000 shares of 250,000 share of Southern Corp for a price of $9,000,000. Southern reported net income on June 30 of $500,000 and paid...

Question 1(15 Marks): A firm produces one good with a technology given by the production function y = f (x) = x1/3. The factor price w and the price p for the good are fixed. a) Explore whether the...

A large country named H is considering an import tariff to protect the industry that produces good X. The world's excess supply curve is P=1000+3X. Country H's excess demand curve is P=8000-50X. a.)...

The Heating Division of Sheffield International produces a heating element that it sells to its customers for $44 per unit. Its unit variable cost is $27, and its unit fixed cost is $5. Top...

Under statistical process control and process capability analysis, if a process is out-of-control and not capable, the logical next step would be to first... blame the operator of the process....

Peterson Furniture Designs is preparing its annual financial statements dated December 31. Ending Inventory Information about the five major Items stocked for regular sale follows: Required: 1-a....

The company pays a stock dividend of 300,000 shares to its shareholders. The market value of the shares is $10 per share and the par value is $1. There are 1,000,000 shares outstanding. How would the...

In Exercises 15 through 30, find the derivative dy/dx. In some of these problems, you may need to use implicit differentiation or logarithmic differentiation. y ex + et -2x 1 + e

Given the initial-value problem y' = 2/t y + t 2 e t , 1 t 2, y(1) = 0, With exact solution y(t) = t 2 (e t e) : a. Use Eulers method with h = 0.1 to approximate the solution, and compare it with...

Repeat Exercise 1 using the Crank-Nicolson Algorithm. Repeat exercise 1

Although q(x)

Assuming a model With V(i) = 2, what is the generalized regression estimator of ty?

Using the data in nhanes.dat, fit a straight line regression model predicting y = waist circumference (variable bmxwaist) from x =thigh circumference (variable bmxthicr). You plotted these data in...

The linearization estimator of V () can be found by the method outlined in Section 9.1 (see Shah et al., 1977). However, the calculations are easier using the DemnatiRao (2004) method discussed in...