The moment generating function G(t) is defined by or where X is a discrete or continuous random
Question:
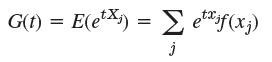
or
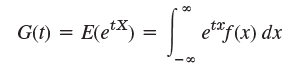
where X is a discrete or continuous random variable, respectively.
(a) Assuming that term wise differentiation and differentiation under the integral sign are permissible, show that E(Xk) = G(k)(0), where G(k) = dkG/dtk, in particular, μ = G'(0).
(b) Prove (4).
(c) Show that the Poisson distribution has the moment generating function G(t) = e-μeμet and prove (6).
(d)
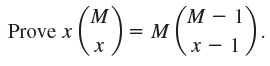
Using this, prove (9).
DistributionThe word "distribution" has several meanings in the financial world, most of them pertaining to the payment of assets from a fund, account, or individual security to an investor or beneficiary. Retirement account distributions are among the most...
Fantastic news! We've Found the answer you've been seeking!
Step by Step Answer:
Related Book For 

Question Posted:





