Consider a small block of mass \(m\) sliding a distance \(s\) down a moving wedge of mass \(M\), as shown in Fig. 14.8. The wedge is also sliding a distance \(x\) along the floor. Neglecting frictional effects, calculate the following:

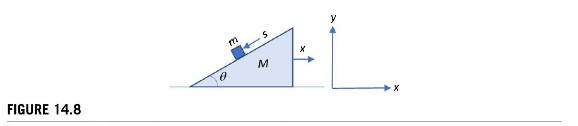
(a) Given that the velocity of the wedge is simply \(\dot{x}\), show that the \(x\) - and \(y\) components of the velocity of the small block are \(v_{x}=\dot{x}-\dot{s} \cos \theta\) and \(v_{y}=-\dot{s} \sin \theta\), respectively.
(b) Show that the total kinetic energy of the combined system is \(T=\frac{1}{2} M \dot{x}^{2}+\) \(\frac{1}{2} m\left(\dot{x}^{2}+\dot{s}^{2}-2 \dot{x} \dot{s} \cos \thetaight)\). Also show that the potential energy for the small block is \(V=-m g s \sin \theta\), where \(g\) is the acceleration due to gravity.
(c) Determine the Euler-Lagrange equations for this problem for the two variables \(s\) and \(x\) and their derivatives.
(d) Solving the Euler-Lagrange equations in part (c), show that the two accelerations \(\ddot{s}\) and \(\ddot{x}\) are given by \[
\ddot{s}=\frac{(M+m)}{\left(M+m \sin ^{2} \thetaight)} g \sin \theta=c_{1} \quad \text { and } \quad \ddot{x}=\frac{M}{\left(M+m \sin ^{2} \thetaight)} g \sin \theta \cos \theta=c_{2} \text {, }
\]
where \(c_{1}\) and \(c_{2}\) are simply constants.

Transcribed Image Text:

FIGURE 14.8 m M X