For Example, 10.3 with = 0, verify that the stresses from equation (10.5.18) reduce to those

For Example, 10.3 with α = 0, verify that the stresses from equation (10.5.18) reduce to those previously given in Eq. (8.4.69).

Data from example 10.3

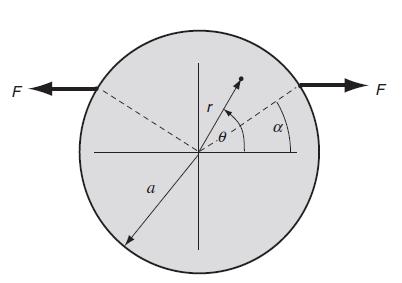
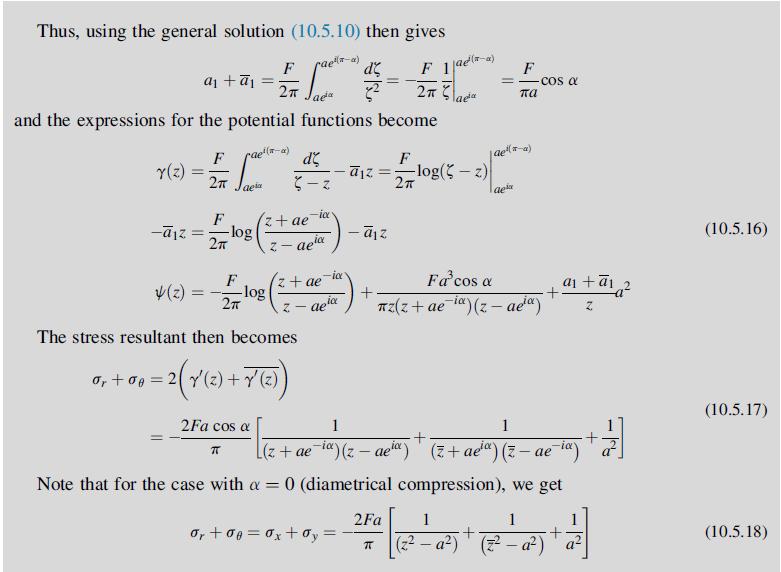
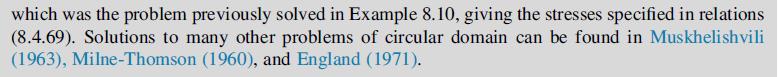
Equation 10.5.18

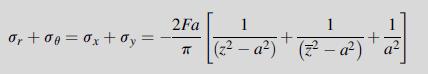
Equation 8.4.69

Transcribed Image Text:

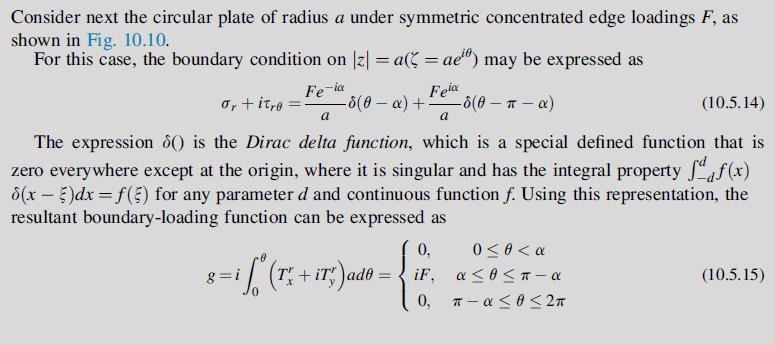
Consider next the circular plate of radius a under symmetric concentrated edge loadings F, as shown in Fig. 10.10. For this case, the boundary condition on |z| = a( = ae) may be expressed as -ia Fe Feia -8(0-) +- -5 (0- - ) a or +itre (10.5.14) a The expression 8() is the Dirac delta function, which is a special defined function that is zero everywhere except at the origin, where it is singular and has the integral property f(x) 8(x - 5)dx = f(5) for any parameter d and continuous function f. Using this representation, the resultant boundary-loading function can be expressed as 0, g=i = i [ ^ (r + iT;) ado = . { / iF, 0, 0 0