Analyze and discuss a limiting case of Prob. 9–99 in which there is no inner cylinder (R_i = ω_i = 0). Generate an expression for u_θ as a function of r. What kind of flow is this? Describe how this flow could be set up experimentally.

Data from Problem 99

Repeat Prob. 9–96 for the more general case. Namely, let the inner cylinder rotate at angular velocity ω_i and let the outer cylinder rotate at angular velocity ω_o. All else is the same as Prob. 9–96. Generate an exact expression for velocity component u_θ as a function of radius r and the other parameters in the problem. Verify that when ω_o = 0 your result simplifies to that of Prob. 9–96.

Data from Problem 96

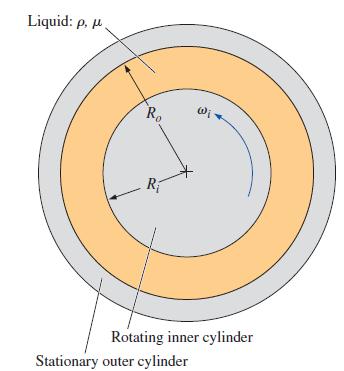
An incompressible Newtonian liquid is confined between two concentric circular cylinders of infinite length— a solid inner cylinder of radius R_i and a hollow, stationary outer cylinder of radius R_o (Fig. P9–96; the z-axis is out of the page). The inner cylinder rotates at angular velocity ω_i. The flow is steady, laminar, and two-dimensional in the r_θ-plane. The flow is also rotationally symmetric, meaning that nothing is a function of coordinate θ (u_θ and P are functions of radius r only). The flow is also circular, meaning that velocity component u_r = 0 everywhere. Generate an exact expression for velocity component u_θ as a function of radius r and the other parameters in the problem. You may ignore gravity.

FIGURE P9–96

Transcribed Image Text:

Liquid: p. p. Ro Wi Rotating inner cylinder Stationary outer cylinder