Figure below shows four cases of flow over the same airfoil, where M gradually increases from 0.3
Question:
Figure below shows four cases of flow over the same airfoil, where M∞ gradually increases from 0.3 to Mcr = 0.61 Point A on the airfoil is the minimum pressure point on the airfoil (and hence the maximum M). Assume that the minimum pressure (Max Mach number) continues to occur at the same point of M∞ increase. In figure (a), for M∞ = 0.3, the local Mach number at point a is arbitrarily chosen to be MA = 0.435. This arbitrariness is reasonable because no airfoil shape is specified, regardless of shape, maximum Mach 0.435 occurs at point A on the airfoil surface. However, once the parameters in (a) are given, then (b), (c), and (d) are not arbitrary. Indeed, Ma is the only unknown function of M∞ in the figure. Taking all of this as background information, starting from the data shown in Figure a, MA is calculated when M∞ = 0.61. Obviously, from figure d, the result should be MA = 1.0 because M∞ = 0.61 is considered Critical Mach number. Which means the Critical Mach number of this airfoil is 0.61. Tip: assume the conditions under the application region of Prandtl-Glauert law. 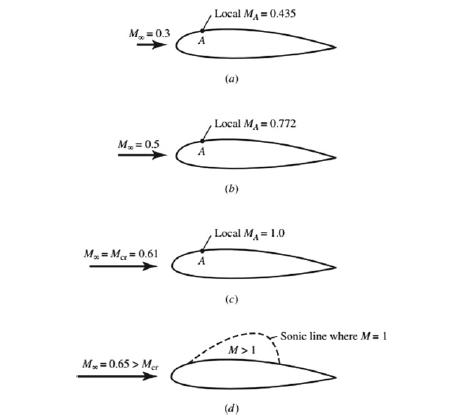
Step by Step Answer:






