Consider the case of a symmetrical double well, such as the one pictured in Figure 9.14. We

Question:

Consider the case of a symmetrical double well, such as the one pictured in Figure 9.14. We are interested in bound states with E < V(0).

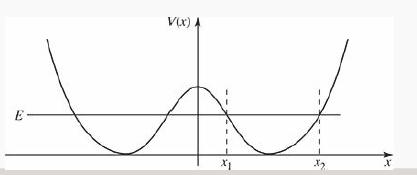
Figure 9.14: Symmetric double well; Problem 9.17.

(a) Write down the WKB wave functions in regions (i) x > x₂, (ii) x₁ < x < x₂, and (iii) 0 < x < x₁. Impose the appropriate connection formulas at x₁and x₂ (this has already been done, in Equation 9.47, for x₂; you will have to work out x₁ for yourself), to show that

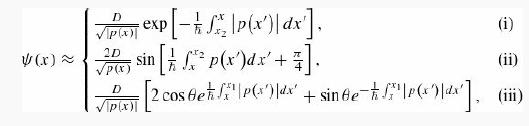
where

(b) Because is symmetric, we need only consider even (+) and odd (-) wave functions. In the former case Ψ'(0) = 0, and in the latter case Ψ(0) = 0. Show that this leads to the following quantization condition

where

Equation 9.60 determines the (approximate) allowed energies
(c) We are particularly interested in a high and/or broad central barrier, in which case ϕ is large, and is huge. Equation 9.60 then tells us that θ
must be very close to a half-integer multiple of π. With this in mind, write θ = (n+1/2)π+∈, where |∈| << 1, and show that the quantization condition becomes

(d) Suppose each well is a parabola

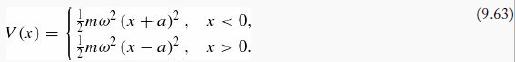
Sketch this potential, find θ (Equation 9.59), and show that

Comment: If the central barrier were impenetrable (∅ → ∞), we would simply have two detached harmonic oscillators, and the energies,
E_n = (n+1/2)ћω, would be doubly degenerate, since the particle could be in the left well or in the right one. When the barrier becomes
finite (putting the two wells into “communication”), the degeneracy is lifted. The even states (Ψ⁺_n) have slightly lower energy, and the odd ones (Ψ^-_n) have slightly higher energy.
(e) Suppose the particle starts out in the right well—or, more precisely, in a state of the form

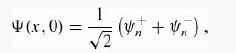
which, assuming the phases are picked in the “natural” way, will be concentrated in the right well. Show that it oscillates back and forth
between the wells, with a period