In this problem we develop time-dependent perturbation theory for a multi-level system, starting with the generalization of Equations 11.5 and 11.6: At time t = 0 we turn on a perturbation H' (t), so that the total Hamiltonian is (a) Generalize Equation 11.10 to read and show that where (b) If the system starts out in the state N

Chapter 11, Problems #24

In this problem we develop time-dependent perturbation theory for a multi-level system, starting with the generalization of Equations 11.5 and 11.6:

At time t = 0 we turn on a perturbation H' (t), so that the total Hamiltonian is

(a) Generalize Equation 11.10 to read

and show that

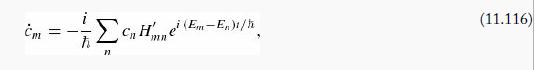
where

(b) If the system starts out in the state Ψ_N, show that (in first-order perturbation theory)

and

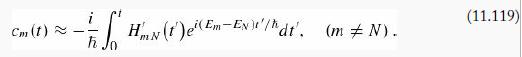
(c) For example, suppose Ĥ' is constant (except that it was turned on at t = 0, and switched off again at some later time . Find the probability of transition from state N to state M (M ≠ N), as a function of T.

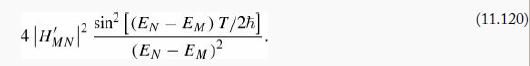
(d) Now suppose Ĥ' is a sinusoidal function of time: Ĥ' = V cos(ωt). Making the usual assumptions, show that transitions occur only to states with energy E_M = E_N ± ћω, and the transition probability is

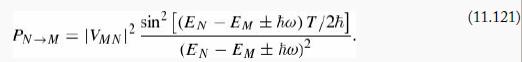
(e) Suppose a multi-level system is immersed in incoherent electromagnetic radiation. Using Section 11.2.3 as a guide, show that the transition rate for stimulated emission is given by the same formula (Equation 11.54) as for a two-level system.