This project is of direct relevance to the concepts of a giant component in Sec. 8.4.
The process consisting of percolation on a lattice is another paradigmatic model, which conceptually is of great relevance to complex systems [84, 263, 424]. Consider a d-dimensional hypercubic lattice of size N = L^d. Assume that a site is occupied (by whatever you fancy: a coin, an elephant, a tree, …) with probability p. On average, the number of occupied sites is N_occ = pN. We define clusters as sets of occupied sites that are connected through nearest-neighbour adjacency. See Fig. 8.17.

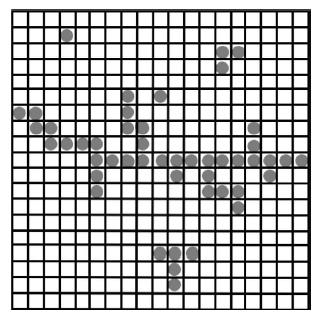This is a purely geometric process. No interaction is assumed between the sites, so in this respect the situation is simpler than in the Ising model in Sec. 6.2.
In the usual spirit of mean-field theory we have the following identity for the probability P_∞ that a randomly selected site belongs to the system-spanning giant cluster:

Here, p = density of occupied site.
• First satisfy yourself that the expression above follows from the fact that:
(1) 1 − P_∞ = probability site doesn’t belong to the system spanning cluster.
(2) pP_∞ = probability that a neighbour site is occupied and belongs to the giant cluster.
(3) For a site not to belong to the giant cluster only, none of its neighbours should be part of the giant cluster.

• Next use the equation to verify that p_c = 1/q_c is a critical value for p, in the sense that for p c we have P_∞ = 0 and for p > p_c we have P_∞ > 0.
• Consider the critical exponent β given by 

P_∞ ∝ (p − p_c)^β
for p in the vicinity above p_c. By expanding Eq. (*) about p_c show that β = 1.