Make the given changes in the indicated examples of this section and then solve the resulting problems.

In Example 3, change the + to − in the second equation and then solve the system of equations.

Data from Example 3

Use the method of elimination by addition or subtraction to solve the system of equations.
x − 3y = 6

2x + 3y = 3

We look at the coefficients to determine the best way to eliminate one of the unknowns. Since the coefficients of the y-terms are numerically the same and opposite in sign, we may immediately add terms of the two equations together to eliminate y. Adding the terms of the left sides and adding terms of the right sides, we obtain

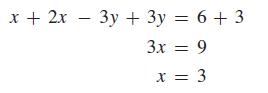
Substituting this value into the first equation, we obtain

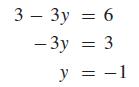
The solution x = 3, y = −1 agrees with the results obtained for the same problem illustrated in Example 1.

x + 2x - 3y + 3y = 6 + 3 +3 3x = 9 x = 3