Consider a log-reciprocal model that relates the logarithm of the dependent variable to the reciprocal of the explanatory variable, \(\ln (y)=\beta_{1}+\beta_{2}(1 / x)\).

a. For what values of \(y\) is this model defined? Are there any values of \(x\) that cause problems?

b. Write the model in exponential form as \(y=\exp \left[\beta_{1}+\beta_{2}(1 / x)\right]\). Show that the slope of this relationship is \(d y / d x=\exp \left[\beta_{1}+\left(\beta_{2} / x\right)\right] \times\left(-\beta_{2} / x^{2}\right)\). What sign must \(\beta_{2}\) have for \(y\) and \(x\) to have a positive relationship, assuming that \(x>0\) ?

c. Suppose that \(x>0\) but it converges toward zero from above. What value does \(y\) converge to? What does \(y\) converge to as \(x\) approaches infinity?

d. Suppose \(\beta_{1}=0\) and \(\beta_{2}=-4\). Evaluate the slope at the \(x\)-values \(0.5,1.0,1.5,2.0,2.5,3.0,3.5,4.0\). As \(x\) increases, is the slope of the relationship increasing or decreasing, or both?

e. Show that the second derivative of the function is

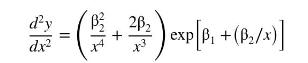
Assuming \(\beta_{2}0\), set the equation to zero, and show that the \(x\)-value that makes the second derivative zero is \(-\beta_{2} / 2\). Does this result agree with your calculations in part (d)?

Transcribed Image Text:

dy B2B dx 202 exp[P + (P/x)]