An alternative definition of Dynkin systems. A family (mathscr{F} subset mathscr{P}(X)) is a Dynkin system if, and

Question:

An alternative definition of Dynkin systems. A family $\mathscr{F} \subset \mathscr{P}(X)$ is a Dynkin system if, and only if,

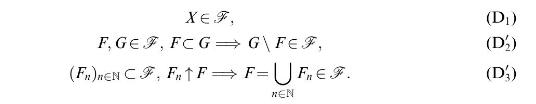
$XEF, F, GEF, FCG G\FEF, (Fn)nN CF, Fn F F=F F. NEN (D) (D) (D3)$

Conclude that any Dynkin system is a monotone class in the sense of Problem 3.14 .

Data from problem 3.14

Monotone classes (1). A family $\mathscr{M} \subset \mathscr{P}(X)$ which contains $X$ and is stable under countable unions of increasing sets and countable intersections of decreasing sets
\[
\begin{array}{ll}
\left(A_{n}ight)_{n \in \mathbb{N}} \subset \mathscr{M}, & A_{1} \subset \cdots \subset A_{n} \subset A_{n+1} \uparrow A=\bigcup_{n \in \mathbb{N}} A_{n} \Longrightarrow A \in \mathscr{M} \\
\left(B_{n}ight)_{n \in \mathbb{N}} \subset \mathscr{M}, & B_{1} \supset \cdots \supset B_{n} \supset B_{n+1} \downarrow B=\bigcap_{n \in \mathbb{N}} B_{n} \Longrightarrow B \in \mathscr{M}
\end{array}
\]
is called a monotone class. Assume that $\mathscr{M}$ is a monotone class and $\mathscr{F} \subset \mathscr{P}(X)$ any family of sets.
(i) Mimic the proof of Theorem 3.4 to show that there is a minimal monotone class $\mathfrak{m}(\mathscr{F})$ such that $\mathscr{F} \subset \mathfrak{m}(\mathscr{F})$.
(ii) If $\mathscr{F}$ is stable w.r.t. complements, i.e. $F \in \mathscr{F} \Longrightarrow F^{c} \in \mathscr{F}$, then so is $\mathfrak{m}(\mathscr{F})$.
(iii) If $\mathscr{F}$ is $\cap$-stable, i.e. $F, G \in \mathscr{F} \Longrightarrow F \cap G \in \mathscr{F}$, then so is $\mathfrak{m}(\mathscr{F})$.
[ show that the families
\[
\begin{aligned}
\Sigma & :=\{M \in \mathfrak{m}(\mathscr{F}): M \cap F \in \mathfrak{m}(\mathscr{F}) \forall F \in \mathscr{F}\} \\
\Sigma^{\prime} & :=\{M \in \mathfrak{m}(\mathscr{F}): M \cap N \in \mathfrak{m}(\mathscr{F}) \forall N \in \mathfrak{m}(\mathscr{F})\}
\end{aligned}
\]
are again monotone classes satisfying $\mathscr{F} \subset \Sigma, \Sigma^{\prime}$.]
(iv) Use (i)-(iii) to prove the following.
Monotone class theorem. Let $\mathscr{F} \subset \mathscr{P}(X)$ be a family of sets which is stable under the formation of complements and intersections. If $\mathscr{M} \supset \mathscr{F}$ is a monotone class, then $\mathscr{M} \supset \sigma(\mathscr{F})$.