Fix an integer 1 and define the Markov chain (Xn)n0 on {, + 1,
Fantastic news! We've Found the answer you've been seeking!
Question:
Fix an integer α ≥ 1 and define the Markov chain (Xn)n≥0 on {α, α + 1, . . .} by the transition probabilities, pi,i+1 = 1 − pi,i−1 = α i , i = α, α + 1, α + 2, . . . Determine the values of α for which the chain is positive recurrent, and for those values, find a stationary distribution of the chain. 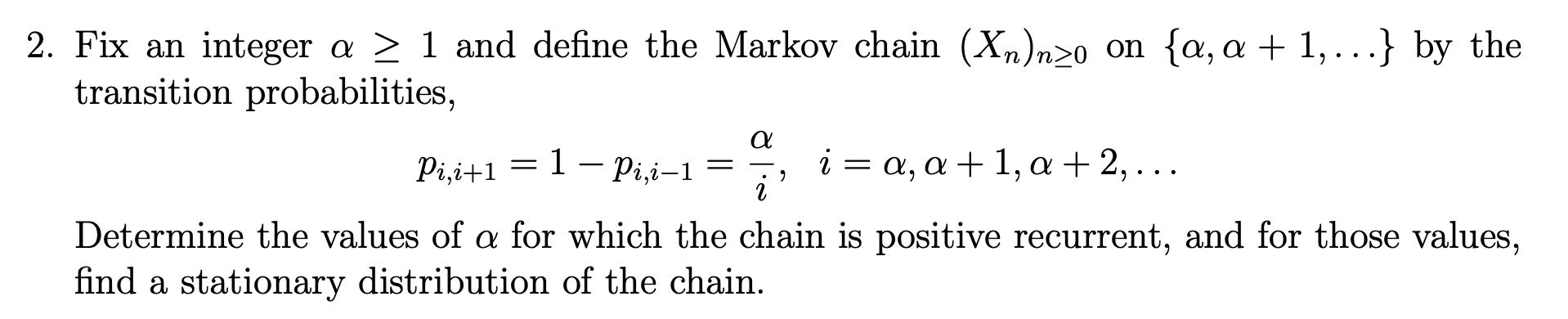
Related Book For 

College Mathematics for Business Economics Life Sciences and Social Sciences
ISBN: 978-0321614001
12th edition
Authors: Raymond A. Barnett, Michael R. Ziegler, Karl E. Byleen
Posted Date:




