Consider absorption into a falling film, where the film contains a reactive component in great excess. This

Question:

Consider absorption into a falling film, where the film contains a reactive component in great excess. This results in reaction that is pseudo-first-order in absorbate concentration. Dilute solutions prevail.

a. Assuming the same simplifications as in Section 10.4, pare down the species continuity equation and determine the partial differential equation governing steady-state operation of the device.

b. What are the boundary conditions?

c. Show that the solution for the concentration profile is:

\[\begin{aligned}\frac{c_{a}}{c_{a o}}= & \frac{1}{2} \exp \left(-y \sqrt{\frac{k^{\prime \prime}}{D_{a b}}}\right) \operatorname{erfc}\left(\frac{y}{\sqrt{4 D_{a b} x / v_{\max }}}-\sqrt{\frac{k^{\prime \prime} v_{\max }}{x}}\right) \\& +\frac{1}{2} \exp \left(-y \sqrt{\frac{k^{\prime \prime}}{D_{a b}}}\right) \operatorname{erfc}\left(\frac{y}{\sqrt{4 D_{a b} x / v_{\max }}}+\sqrt{\frac{k^{\prime \prime} v_{\max }}{x}}\right)\end{aligned}\]

d. Show that the molar flux of \(a\) at the interface, \(y=0\), is:
\[N_{a}=c_{a o} \sqrt{D_{a b} k^{\prime \prime}}\left[\operatorname{erf}\left(\sqrt{\frac{k^{\prime \prime} x}{v_{\max }}}\right)+\frac{\exp \left(-\frac{k^{\prime \prime} x}{v_{\max }}\right)}{\sqrt{\frac{\pi k^{\prime \prime} x}{v_{\max }}}}\right]\]

e. What is the mass transfer coefficient for this system?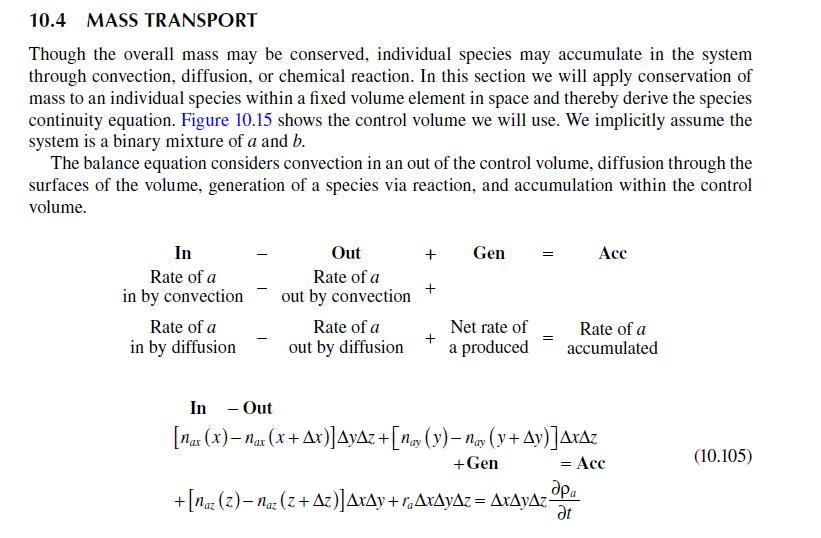 image text in transcribed